Article contents
SURREAL ORDERED EXPONENTIAL FIELDS
Published online by Cambridge University Press: 13 August 2021
Abstract
In 2001, the algebraico-tree-theoretic simplicity hierarchical structure of J. H. Conway’s ordered field  ${\mathbf {No}}$ of surreal numbers was brought to the fore by the first author and employed to provide necessary and sufficient conditions for an ordered field (ordered
${\mathbf {No}}$ of surreal numbers was brought to the fore by the first author and employed to provide necessary and sufficient conditions for an ordered field (ordered 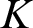 $K$-vector space) to be isomorphic to an initial subfield (
$K$-vector space) to be isomorphic to an initial subfield (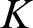 $K$-subspace) of
$K$-subspace) of  ${\mathbf {No}}$, i.e. a subfield (
${\mathbf {No}}$, i.e. a subfield (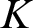 $K$-subspace) of
$K$-subspace) of  ${\mathbf {No}}$ that is an initial subtree of
${\mathbf {No}}$ that is an initial subtree of  ${\mathbf {No}}$. In this sequel, analogous results are established for ordered exponential fields, making use of a slight generalization of Schmeling’s conception of a transseries field. It is further shown that a wide range of ordered exponential fields are isomorphic to initial exponential subfields of
${\mathbf {No}}$. In this sequel, analogous results are established for ordered exponential fields, making use of a slight generalization of Schmeling’s conception of a transseries field. It is further shown that a wide range of ordered exponential fields are isomorphic to initial exponential subfields of  $({\mathbf {No}}, \exp )$. These include all models of
$({\mathbf {No}}, \exp )$. These include all models of 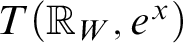 $T({\mathbb R}_W, e^x)$, where
$T({\mathbb R}_W, e^x)$, where 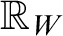 ${\mathbb R}_W$ is the reals expanded by a convergent Weierstrass system W. Of these, those we call trigonometric-exponential fields are given particular attention. It is shown that the exponential functions on the initial trigonometric-exponential subfields of
${\mathbb R}_W$ is the reals expanded by a convergent Weierstrass system W. Of these, those we call trigonometric-exponential fields are given particular attention. It is shown that the exponential functions on the initial trigonometric-exponential subfields of  ${\mathbf {No}}$, which includes
${\mathbf {No}}$, which includes  ${\mathbf {No}}$ itself, extend to canonical exponential functions on their surcomplex counterparts. The image of the canonical map of the ordered exponential field
${\mathbf {No}}$ itself, extend to canonical exponential functions on their surcomplex counterparts. The image of the canonical map of the ordered exponential field 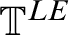 ${\mathbb T}^{LE}$ of logarithmic-exponential transseries into
${\mathbb T}^{LE}$ of logarithmic-exponential transseries into  ${\mathbf {No}}$ is shown to be initial, as are the ordered exponential fields
${\mathbf {No}}$ is shown to be initial, as are the ordered exponential fields 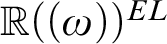 ${\mathbb R}((\omega ))^{EL}$ and
${\mathbb R}((\omega ))^{EL}$ and  ${\mathbb R}\langle \langle \omega \rangle \rangle $.
${\mathbb R}\langle \langle \omega \rangle \rangle $.
Information
- Type
- Article
- Information
- Copyright
- © Association for Symbolic Logic 2021
Footnotes
The in-text reference citations have been corrected. An erratum detailing this update has also been published (doi: 10.1017/jsl.2022.5).
References
REFERENCES
A correction has been issued for this article:
- 5
- Cited by
Linked content
Please note a has been issued for this article.


