Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-25T18:45:27.840Z Has data issue: false hasContentIssue false
  • English
  • Français

An incomplete decidable modal logic

Published online by Cambridge University Press:  12 March 2014

M. J. Cresswell
M. J. Cresswell*
Affiliation:
Victoria University of Wellington, Wellington, New Zealand
Get access Rights & Permissions [Opens in a new window]

Extract

The most common way of proving decidability in propositional modal logic is to shew that the system in question has the finite model property. This is not however the only way. Gabbay in [4] proves the decidability of many modal systems using Rabin's result in [8] on the decidability of the second-order theory of successor functions. In particular [4, pp. 258-265] he is able to prove the decidability of a system which lacks the finite model property. Gabbay's system is however complete, in the sense of being characterized by a class of frames, and the question arises whether there is a decidable modal logic which is not complete. Since no incomplete modal logic has the finite model property [9, p. 33], any proof of decidability must employ some such method as Gabbay's. In this paper I use the Gabbay/Rabin technique to prove the decidability of a finitely axiomatized normal modal propositional logic which is not characterized by any class of frames. I am grateful to the referee for suggesting improvements in substance and presentation.

The terminology I am using is standard in modal logic. By a frame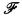 is understood a pair 〈W, R〉 in which W is a class (of “possible worlds”) and R ⊆ W2. To avoid confusion in what follows, a frame will henceforth be referred to as a Kripke frame. By contrast, a general frame is a pair 〈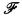, Π〉 in which 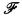is a Kripke frame and Π is a collection of subsets of W closed under the Boolean operations and satisfying the condition that if A is in Π then so is R−1 “A. A model on a frame (of either kind) is obtained by adding a function V which assigns sets of worlds to propositional variables. In the case of a general frame we require that V(p) ∈ Π.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 49 , Issue 2 , June 1984 , pp. 520 - 527
Copyright
Copyright © Association for Symbolic Logic 1984

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]van Benthem, J. F. A. A., Syntactic aspects of modal incompleteness theorems, Theoria, vol. 45 (1979), pp. 63–77.CrossRefGoogle Scholar
[2]Boolos, G., The unprovability of consistency, Cambridge University Press, Cambridge, 1979.Google Scholar
[3]Fine, K., An incomplete logic containing S4, Theoria, vol. 40 (1974), pp. 23–29.CrossRefGoogle Scholar
[4]Gabbay, D. M., Investigations in modal and tense logics with applications to problems in philosophy and linguistics, Reidel, Dordrecht, 1976.CrossRefGoogle Scholar
[5]Hughes, G. E. and Cresswell, , An introduction to modal logic, Methuen, London, 1968.Google Scholar
[6]Lemmon, E. J., The “Lemmon notes”: An introduction to modal logic (Segerberg, K., editor), Blackwell, Oxford, 1977.Google Scholar
[7]Makinson, D. C., A generalization of the concept of a relational model for modal logic, Theoria, vol. 36(1970), pp. 330–335.CrossRefGoogle Scholar
[8]Rabin, M. O., Decidability of some second-order theories and automata on infinite trees, Transactions of the American Mathematical Society, vol. 141 (1969), pp. 1–35.Google Scholar
[9]Segerberg, K., An essay in classical modal logic, Department of Philosphy, Uppsala University, Uppsala, 1971.Google Scholar
[10]Thomason, S. K., Semantic analysis of tense logics, this Journal, vol. 37 (1972), pp. 150–158.Google Scholar
[11]Thomason, S. K., An incompleteness theorem in modal logic, Theoria, vol. 40 (1974), pp. 30–34.CrossRefGoogle Scholar