Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-25T18:31:32.883Z Has data issue: false hasContentIssue false
  • English
  • Français

Analytic equivalence relations and bi-embeddability

Published online by Cambridge University Press:  12 March 2014

Sy-David Friedman  and
Luca Motto Ros
Sy-David Friedman
Affiliation:
Kurt Gödel Research Center for Mathematical Logic, University of Vienna, Wahringer Straße 25, A-1090 Vienna, Austria, E-mail: sdf@logic.univie.ac.at
Luca Motto Ros
Affiliation:
Kurt Gödel Research Center for Mathematical Logic, University of Vienna, Wahringer Straße 25, A-1090 Vienna, Austria, E-mail: luca.mottoros@libero.it
Get access Rights & Permissions [Opens in a new window]

Abstract

Louveau and Rosendal [5] have shown that the relation of bi-embeddability for countable graphs as well as for many other natural classes of countable structures is complete under Borel reducibility for analytic equivalence relations. This is in strong contrast to the case of the isomorphism relation, which as an equivalence relation on graphs (or on any class of countable structures consisting of the models of a sentence of 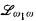) is far from complete (see [5, 2]).

In this article we strengthen the results of [5] by showing that not only does bi-embeddability give rise to analytic equivalence relations which are complete under Borel reducibility, but in fact any analytic equivalence relation is Borel equivalent to such a relation. This result and the techniques introduced answer questions raised in [5] about the comparison between isomorphism and bi-embeddability. Finally, as in [5] our results apply not only to classes of countable structures defined by sentences of 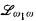, but also to discrete metric or ultrametric Polish spaces, compact metrizable topological spaces and separable Banach spaces, with various notions of embeddability appropriate for these classes, as well as to actions of Polish monoids.

Keywords

Analytic equivalence relationanalytic quasi-orderbi-embeddabilityBorel reducibility
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 76 , Issue 1 , March 2011 , pp. 243 - 266
Copyright
Copyright © Association for Symbolic Logic 2011

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Camerlo, R., Universal analytic preorders arising from surjective functions, Fundamenta Mathematics, vol. 187 (2005), pp. 193–212.CrossRefGoogle Scholar
[2]Friedman, H. and Stanley, L., A Borel reducibility theory for classes of countable structures, this Journal, vol. 54 (1989), no. 3, pp. 894–914.Google Scholar
[3]Hjorth, G., Classification and orbit equivalence relations, Mathematical Surveys and Monographs, vol. 75, American Mathematical Society, Providence, RI, 2000.Google Scholar
[4]Kechris, A. S., Classical descriptive set theory, Graduate Texts in Mathematics, vol. 156, Springer-Verlag, Heidelberg, 1995.CrossRefGoogle Scholar
[5]Louveau, A. and Rosendal, C., Complete analytic equivalence relations, Transactions of the American Mathematical Society, vol. 357 (2005), no. 12, pp. 4839–4866.CrossRefGoogle Scholar
[6]Marcone, A. and Rosendal, C., The complexity of continuous embeddability between dendrites, this Journal, vol. 69 (2004), no. 3, pp. 663–673.Google Scholar
[7]Miller, A. W., Vaught's conjecture for theories of one unary operation, Polska Akademia Nauk. Fundamenta Mathematicae, vol. 111 (1981), no. 2, pp. 135–141.CrossRefGoogle Scholar
[8]Moschovakis, Y. N., Descriptive set theory, Studies in Logic and the Foundations of Mathematics, vol. 100, North-Holland, Amsterdam, 1980.Google Scholar
[9]Rubin, M., Theories of linear order, Israel Journal of Mathematics, vol. 17 (1974), pp. 392–443.CrossRefGoogle Scholar
[10]Rubin, M., Vaught's conjecture for linear orderings, Notices of American Mathematical Society, vol. 24(1977), pp. A–390.Google Scholar
[11]Steel, J., On Vaught's conjecture. Cabal seminar 76-77, Proceedings of the Caltech-UCLA Logic Seminar, Springer-Verlag, 1976–1977.Google Scholar
[12]Boom, M. Vanden, The effective Borel hierarchy, Fundamenta Mathematicae, vol. 195 (2007), pp. 269–289.CrossRefGoogle Scholar