Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Lange, Karen
and
Soare, Robert I.
2007.
Computability of Homogeneous Models.
Notre Dame Journal of Formal Logic,
Vol. 48,
Issue. 1,
Fokina, Ekaterina B.
Harizanov, Valentina
and
Melnikov, Alexander
2014.
Turing's Legacy.
p.
124.

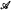 , i.e., the elementary diagram
, i.e., the elementary diagram 