Co-critical points of elementary embeddings

Extract

Probably the two most famous examples of elementary embeddings between inner models of set theory are the embeddings of the universe into an inner model given by a measurable cardinal and the embeddings of the constructible universe L into itself given by 0^#. In both of these examples, the “target model” is a subclass of the “ground model” (and in the latter case they are equal). It is not hard to find examples of embeddings in which the target model is not a subclass of the ground model: if 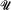is a generic ultrafilter arising from forcing with a precipitous ideal on a successor cardinal κ, then the ultraproduct of the ground model via 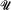collapses κ. Such considerations suggest a classification of how close the target model comes to “fitting inside” the ground model.

Definition 1.1. Let M and N be inner models (transitive, proper class models) of ZFC, and let j: M → N be an elementary embedding. The co-critical point of j is the least ordinal λ, if any exist, such that there is X ⊆ λ, X ∈ N but X ∉ M. Such an X is called a new subset of λ.

It is easy to see that the co-critical point of j: M → N is a cardinal in N.