No CrossRef data available.
Article contents
Conditioned disjunction as a primitive connective for the erweiterter Aussagenkalkül
Published online by Cambridge University Press: 12 March 2014
Extract
It has been shown that the conditioned disjunction function [X, Y, Z] with the same truth-table as (X & Y) ∨ (Z & 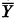 ) together with the logical constants t and f, form a complete set of independent connectives for the 2-valued propositional calculus and that these connectives are self-dual. This has since been generalised to the theorem which states that the conditioned disjunction function [Y, X1, X2, …, Xm, Y] with the same truth-table as (X1 & J1(Y)) ∨ (X2 & J2(Y)) ∨ … ∨ (Xm & Jm(Y)) together with the logical constants 1, 2, …, m form a complete set of independent connectives for the m-valued propositional calculus and that these connectives are self-dual. It has been conjectured by Church that conditioned disjunction together with the universal and existential quantifiers form a complete set of independent connectives for the 2-valued erweiterter Aussagenkalkül. The object of the present paper is to prove a theorem for the m-valued erweiterter Aussagenkalkül which reduces, in the case m = 2, to the conjecture of Church. In the m-valued propositional calculus if the propositional variable X occurs as a free variable in the formula
) together with the logical constants t and f, form a complete set of independent connectives for the 2-valued propositional calculus and that these connectives are self-dual. This has since been generalised to the theorem which states that the conditioned disjunction function [Y, X1, X2, …, Xm, Y] with the same truth-table as (X1 & J1(Y)) ∨ (X2 & J2(Y)) ∨ … ∨ (Xm & Jm(Y)) together with the logical constants 1, 2, …, m form a complete set of independent connectives for the m-valued propositional calculus and that these connectives are self-dual. It has been conjectured by Church that conditioned disjunction together with the universal and existential quantifiers form a complete set of independent connectives for the 2-valued erweiterter Aussagenkalkül. The object of the present paper is to prove a theorem for the m-valued erweiterter Aussagenkalkül which reduces, in the case m = 2, to the conjecture of Church. In the m-valued propositional calculus if the propositional variable X occurs as a free variable in the formula 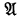 then (∃X)
then (∃X)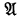 and (X)
and (X)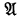 are read “there exists X such that
are read “there exists X such that 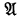 ” and “for all X,
” and “for all X, 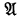 ”, respectively. If for a given assignment of truth-values to the remaining free propositional variables occurring in
”, respectively. If for a given assignment of truth-values to the remaining free propositional variables occurring in 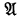 ,
, 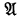 takes the truth-value f(x), where x is the truth-value of X, then (∃X)
takes the truth-value f(x), where x is the truth-value of X, then (∃X)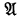 and (X)
and (X)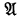 take the truth-values min (f(1), f(2), …, f(m)), max(f(1), f(2), …, f(m)), respectively. We shall prove:
take the truth-values min (f(1), f(2), …, f(m)), max(f(1), f(2), …, f(m)), respectively. We shall prove:
Theorem. The conditioned disjunction function, together with the universal and existential quantifiers, form a complete set of independent connectives for the m-valued erweiterter Aussagenkalkül.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1953
References
1 Church, Alonzo, Conditioned disjunction as a primitive connective for the prepositional calculus, Portugaliae Mathematica, vol. 7 (1948), pp. 87–90Google Scholar.
2 I.e. given any truth-table, a function with that truth-table can be defined in terms of the primitives, but if any one of the primitives is omitted there are functions which cannot be defined in terms of the remaining primitives.
3 Rose, Alan, Conditioned disjunction as a primitive connective for the m-valued prepositional calculus, Mathematische Annalen, vol. 123 (1951), pp. 76–78CrossRefGoogle Scholar.
4 The J-functions are those used by Rosser, J. B. and Turquette, A. R. in their paper Axiom schemes for m-valued prepositional calculi, this Journal, vol. 10 (1945), pp. 61–82Google Scholar.
5 Op. cit.
6 See Łukasiewicz, Jan and Tarski, Alfred, Untersuchungen über den Aussagenkalkül, Comptes rendus des séances de la Société des Sciences et des Lettres de Varsovie, Classe III, vol. 23 (1930), pp. 30–50Google Scholar; also Russell, B., The theory of implication, American Journal of Mathematics, vol. 28 (1906), pp. 159–202CrossRefGoogle Scholar.
7 It may be necessary first to rename some of the bound variables occurring in ![]() i, in order to avoid collision between free and bound variables.
i, in order to avoid collision between free and bound variables.
8 If ![]() always takes the same truth-value as Ψ(X 1, X 2, …, Xn), where the symbol “−” denotes the negation function of Łukasiewicz (op. cit.), then Φ is said to be the dual of Ψ.
always takes the same truth-value as Ψ(X 1, X 2, …, Xn), where the symbol “−” denotes the negation function of Łukasiewicz (op. cit.), then Φ is said to be the dual of Ψ.

