Article contents
Constructible falsity and inexact predicates
Published online by Cambridge University Press: 12 March 2014
Extract
In 1949 Nelson [5] proposed a constructive logic in which falsity is conceived in a fashion analogous to that for intuitionistic truth. The predicate calculus N (for strong negation) was characterized by the usual axioms and rules for positive intuitionistic connectives (see Kleene [4, p. 82, la–7 and 9–12]), with the additional axiom schemata for strong negation: A ⊃ (¬A ⊃ B),
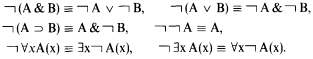
Nelson's paper showed that N may be interpreted with concepts for constructive truth (P-realizability) and falsity (N-realizability). The paper also gave mappings between the intuitionistic and strong negation systems of arithmetic.
These mappings are easily adapted to pure predicate calculus. One shows that intuitionistic predicate calculus I is a subsystem of N by reading the intuitionistic negation of A as A ⊃ B & ¬B in N. It is possible to map N into a subsystem of I using the definition of A′ in [5, p. 19] changing only the definition of (¬A)′ for atomic A to A ⊃ B & ¬B.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1984
References
REFERENCES
- 198
- Cited by

