Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Soskova, Mariya I.
2013.
The Nature of Computation. Logic, Algorithms, Applications.
Vol. 7921,
Issue. ,
p.
371.
Ganchev, Hristo
and
Soskova, Mariya
2014.
Definability via Kalimullin pairs in the structure of the enumeration degrees.
Transactions of the American Mathematical Society,
Vol. 367,
Issue. 7,
p.
4873.
Soskova, Mariya I.
2016.
The automorphism group of the enumeration degrees.
Annals of Pure and Applied Logic,
Vol. 167,
Issue. 10,
p.
982.
Cai, Mingzhong
Lempp, Steffen
Miller, Joseph S.
and
Soskova, Mariya I.
2016.
On Kalimullin pairs.
Computability,
Vol. 5,
Issue. 2,
p.
111.
2016.
Algebraic Computability and Enumeration Models.
p.
155.
Ganchev, Hristo
and
Skordev, Dimiter
2017.
The Incomputable.
p.
11.
Ganchev, Hristo A.
Soskova, Mariya I.
and
Löwe, Benedikt
2018.
The jump hierarchy in the enumeration degrees.
Computability,
Vol. 7,
Issue. 2-3,
p.
179.
GANCHEV, HRISTO A.
KALIMULLIN, ISKANDER SH.
MILLER, JOSEPH S.
and
SOSKOVA, MARIYA I.
2022.
A STRUCTURAL DICHOTOMY IN THE ENUMERATION DEGREES.
The Journal of Symbolic Logic,
Vol. 87,
Issue. 2,
p.
527.

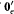 in the local structure of the enumeration degrees,
in the local structure of the enumeration degrees,  , contains at least one low-cuppable member. We apply this new structural property to show that the classes of all
, contains at least one low-cuppable member. We apply this new structural property to show that the classes of all 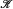 -pairs in
-pairs in 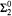 enumeration degrees and all upwards properly
enumeration degrees and all upwards properly 