Hostname: page-component-cd9895bd7-hc48f Total loading time: 0 Render date: 2024-12-25T18:11:54.640Z Has data issue: false hasContentIssue false
  • English
  • Français

DEGREES OF CATEGORICITY AND SPECTRAL DIMENSION

Published online by Cambridge University Press:  01 May 2018

NIKOLAY A. BAZHENOV ,
ISKANDER SH. KALIMULLIN  and
MARS M. YAMALEEV
NIKOLAY A. BAZHENOV
Affiliation:
LABORATORY OF COMPUTABILITY THEORY AND APPLIED LOGIC SOBOLEV INSTITUTE OF MATHEMATICS PR. AKAD. KOPTYUGA 4 NOVOSIBIRSK 630090, RUSSIA and DEPARTMENT OF MATHEMATICS AND MECHANICS NOVOSIBIRSK STATE UNIVERSITY UL. PIROGOVA 2 NOVOSIBIRSK 630090, RUSSIA E-mail: bazhenov@math.nsc.ru
ISKANDER SH. KALIMULLIN
Affiliation:
N.I. LOBACHEVSKY INSTITUTE OF MATHEMATICS AND MECHANICS KAZAN FEDERAL UNIVERSITY UL. KREMLEVSKAYA 18 KAZAN 420008, RUSSIA E-mail: iskander.kalimullin@kpfu.ru
MARS M. YAMALEEV
Affiliation:
N.I. LOBACHEVSKY INSTITUTE OF MATHEMATICS AND MECHANICS KAZAN FEDERAL UNIVERSITY UL. KREMLEVSKAYA 18 KAZAN 420008, RUSSIA E-mail: mars.yamaleev@kpfu.ru
Get access Rights & Permissions [Opens in a new window]

Abstract

A Turing degree d is the degree of categoricity of a computable structure ${\cal S}$ if d is the least degree capable of computing isomorphisms among arbitrary computable copies of ${\cal S}$. A degree d is the strong degree of categoricity of ${\cal S}$ if d is the degree of categoricity of ${\cal S}$, and there are computable copies 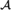${\cal A}$ and ${\cal B}$ of ${\cal S}$ such that every isomorphism from 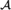${\cal A}$ onto ${\cal B}$ computes d. In this paper, we build a c.e. degree d and a computable rigid structure 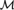${\cal M}$ such that d is the degree of categoricity of 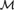${\cal M}$, but d is not the strong degree of categoricity of 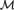${\cal M}$. This solves the open problem of Fokina, Kalimullin, and Miller [13].

For a computable structure ${\cal S}$, we introduce the notion of the spectral dimension of ${\cal S}$, which gives a quantitative characteristic of the degree of categoricity of ${\cal S}$. We prove that for a nonzero natural number N, there is a computable rigid structure 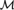${\cal M}$ such that 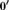$0\prime$ is the degree of categoricity of 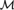${\cal M}$, and the spectral dimension of 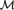${\cal M}$ is equal to N.

Keywords

03D4503C57categoricity spectrumdegree of categoricityrigid structurecomputable categoricity
Type
Articles
Information
The Journal of Symbolic Logic , Volume 83 , Issue 1 , March 2018 , pp. 103 - 116
Copyright
Copyright © The Association for Symbolic Logic 2018 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Anderson, B. A. and Csima, B. F., Degrees that are not degrees of categoricity. Notre Dame Journal of Formal Logic, vol. 57 (2016), no. 3, pp. 289–398.Google Scholar
Ash, C. J., Recursive labelling systems and stability of recursive structures in hyperarithmetical degrees. Transactions of the American Mathematical Society, vol. 298 (1986), pp. 497–514.Google Scholar
Ash, C. J., Stability of recursive structures in arithmetical degrees. Annals of Pure and Applied Logic, vol. 32 (1986), pp. 113–135.Google Scholar
Ash, C. J. and Knight, J. F., Computable Structures and the Hyperarithmetical Hierarchy, Studies in Logic and the Foundations of Mathematics, vol. 144, Elsevier Science B.V., Amsterdam, 2000.Google Scholar
Bazhenov, N. A., Degrees of categoricity for superatomic Boolean algebras. Algebra Logic, vol. 52 (2013), no. 3, pp. 179–187.Google Scholar
Bazhenov, N. A., ${\rm{\Delta }}_2^0$-categoricity of Boolean algebras. Journal of Mathematical Sciences, vol. 203 (2014), no. 4, pp. 444–454.Google Scholar
Bazhenov, N. A., Autostability spectra for Boolean algebras. Algebra Logic, vol. 53 (2015), no. 6, pp. 502–505.Google Scholar
Bazhenov, N. A., Kalimullin, I. S., and Yamaleev, M. M., Degrees of categoricity vs. strong degrees of categoricity. Algebra Logic, vol. 55 (2016), no. 2, pp. 173–177.Google Scholar
Csima, B. F., Franklin, J. N. Y., and Shore, R. A., Degrees of categoricity and the hyperarithmetic hierarchy. Notre Dame Journal of Formal Logic, vol. 54 (2013), no. 2, pp. 215–231.Google Scholar
Csima, B. F. and Stephenson, J., Finite computable dimension and degrees of categoricity, to appear. Available at www.math.uwaterloo.ca/∼csima/papers/finitecompdemdegcat.pdf.Google Scholar
Ershov, Y. L. and Goncharov, S. S., Constructive Models, Kluwer Academic/Plenum Publishers, New York, 2000.Google Scholar
Fokina, E., Frolov, A., and Kalimullin, I., Categoricity spectra for rigid structures. Notre Dame Journal of Formal Logic, vol. 57 (2016), no. 1, 45–57.Google Scholar
Fokina, E. B., Kalimullin, I., and Miller, R., Degrees of categoricity of computable structures. Archive for Mathematical Logic, vol. 49 (2010), no. 1, pp. 51–67.Google Scholar
Frolov, A. N., Effective categoricity of computable linear orderings. Algebra Logic, vol. 54 (2015), no. 5, pp. 415–417.Google Scholar
Fröhlich, A. and Shepherdson, J. C., Effective procedures in field theory. Philosophical transactions of the Royal Society of London, Series A, vol. 248 (1956), 950, pp. 407–432.Google Scholar
Goncharov, S. S., Degrees of autostability relative to strong constructivizations. Proceedings of the Steklov Institute of Mathematics, vol. 274 (2011), pp. 105–115.Google Scholar
Hirschfeldt, D. R., Khoussainov, B., Shore, R. A., and Slinko, A. M., Degree spectra and computable dimensions in algebraic structures. Annals of Pure and Applied Logic, vol. 115 (2002), no. 1–3, pp. 71–113.Google Scholar
Mal’tsev, A. I., Constructive algebras. I. Russian Mathematical Surveys, vol. 16 (1961), no. 3, pp. 77–129.Google Scholar
Mal’tsev, A. I., On recursive abelian groups. Soviet Mathematics - Doklady, vol. 32 (1962), pp. 1431–1434.Google Scholar
Miller, R., d-computable categoricity for algebraic fields, this Journal, vol. 74 (2009), no. 4, pp. 1325–1351.Google Scholar
Miller, R. and Shlapentokh, A., Computable categoricity for algebraic fields with splitting algorithms. Transactions of the American Mathematical Society, vol. 367 (2015), no. 6, pp. 3955–3980.Google Scholar