Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Pila, J.
and
Wilkie, A. J.
2006.
The rational points of a definable set.
Duke Mathematical Journal,
Vol. 133,
Issue. 3,
Mamino, Marcello
2014.
On the computing power of +, -, and ×.
p.
1.
Binyamini, Gal
2022.
Point counting for foliations over number fields.
Forum of Mathematics, Pi,
Vol. 10,
Issue. ,
Wilkie, Alex J.
2024.
Analytic continuation and Zilber’s quasiminimality
conjecture.
Model Theory,
Vol. 3,
Issue. 2,
p.
701.
Bhardwaj, Neer
McCulloch, Raymond
Ramachandran, Nandagopal
and
Woo, Katharine
2025.
INTEGER-VALUED O-MINIMAL FUNCTIONS.
The Quarterly Journal of Mathematics,

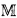 be an o-minimal expansion of the ordered field of real numbers
be an o-minimal expansion of the ordered field of real numbers 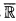 , and let
, and let 