Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-25T19:50:27.843Z Has data issue: false hasContentIssue false
  • English
  • Français

The elementary theory of free pseudo p-adically closed fields of finite corank

Published online by Cambridge University Press:  12 March 2014

Ido Efrat
Ido Efrat*
Affiliation:
School of Mathematical Sciences, Raymond and Beverly Sackler Faculty of Exact Sciences, Tel Aviv University, Ramat Aviv, Tel Aviv 69978, Israel
Get access Rights & Permissions [Opens in a new window]

Extract

Let 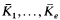be p-adic closures of a countable Hilbertian field K. The main result of [EJ] asserts that the field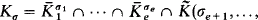 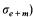has the following properties for almost all σ1,…,σe + m ϵ G(K) (in the sense of the unique Haar measure on G(K)e+m):

(a) Kσ is pseudo p-adically closed (abbreviation: PpC), i.e., each nonempty absolutely irreducible variety defined over Kσ has a Kσ-rational point, provided that it has a simple rational point in each p-adic closure of Kσ.

(b) G(Kσ) ≅ De,m, where De,m is the free profinite product 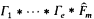of e copies Γ1,…, Γe of G(ℚp) and a free profinite group 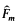of rank m.

(c) Kσ has exactly e nonequivalent p-adic valuation rings. They are the restrictions Oσ1,…, Oσe of the unique p-adic valuation rings on , respectively.

In this paper we show that this result is in a certain sense the best possible. More precisely, we first show that the class of fields which satisfy (a)–(c) above is elementary in the appropriate language 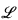e(K), which is the ordinary first-order language of rings augmented by constant symbols for the elements of K and by e new unary relation symbols 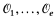(interpreted as e p-adic valuation rings).

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 56 , Issue 2 , June 1991 , pp. 484 - 496
Copyright
Copyright © Association for Symbolic Logic 1991

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[C]Cohen, P. J., Decision procedures for real and p-adic fields, Communications on Pure and Applied Mathematics, vol. 22 (1969), pp. 131–151.CrossRefGoogle Scholar
[EJ]Efrat, I. and Jarden, M., Free pseudo p-adically closed fields of finite corank, Journal of Algebra, vol. 133 (1990), pp. 132–150.CrossRefGoogle Scholar
[FJ]Fried, M. and Jarden, M., Field arithmetic, Springer-Verlag, Berlin, 1986.CrossRefGoogle Scholar
[HJ1]Haran, D. and Jarden, M., The absolute Galois group of a pseudo real closed field, Annali delta Scuola Nor male Superiore di Pisa, ser. 4, vol. 12 (1985), pp. 449–489.Google Scholar
[HJ2]Haran, D., The absolute Galois group of a pseudo p-adically closed field, Journal für die Reine und Angewandte Mathematik, vol. 383 (1988), pp. 147–206.Google Scholar
[J1]Jarden, M., The elementary theory of large e-fold ordered fields, Acta Mathematica, vol. 149 (1982), pp. 239–260.CrossRefGoogle Scholar
[J2]Jarden, M., Algebraic realization of p-adically projective groups, Compositio Mathematica (to appear).Google Scholar
[JK]Jarden, M. and Kiehne, U., The elementary theory of algebraic fields of finite corank, Inventiones Mathematicae, vol. 30 (1975), pp. 275–294.CrossRefGoogle Scholar
[M]Macintyre, A., On definable subsets of p-adic fields, this Journal, vol. 41 (1976), pp. 605–611.Google Scholar
[PR]Prestel, A. and Roquette, P., Formally p-adic fields, Lecture Notes in Mathematics, vol. 1050, Springer-Verlag, Berlin, 1984.CrossRefGoogle Scholar
[S]Serre, J.-P., Cohomologie galoisienne, Lecture Notes in Mathematics, vol. 5, Springer-Verlag, Berlin, 1965.CrossRefGoogle Scholar