Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-25T18:26:42.003Z Has data issue: false hasContentIssue false
  • English
  • Français

A generalization of forking

Published online by Cambridge University Press:  12 March 2014

Siu-Ah Ng
Siu-Ah Ng*
Affiliation:
Department of Pure Mathematics, University of Hull, Hull HU6 7RX, England
Get access Rights & Permissions [Opens in a new window]

Extract

Given a subset A of a fixed saturated model , we let 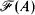denote the algebra of definable subsets of the domain of M of with parameters from A. Then a complete type p over A can be regarded as a measure on 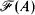, assigning the value 1 to members of p and 0 to nonmembers. In [5] and [6], Keisler developed a theory of forking concerning probability measures. Therefore it generalizes the ordinary theory. On a different track, we can view the complement of the type p, or the collection of null sets of any measure on 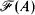, as ideals on 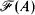. Moreover, ideals and the pseudometric of a measure form examples of the so-called FN topology. Roughly speaking, an FN topology is one that topologizes the ring structure of a Boolean algebra. Associated with this topology is a special basis, called an FN basis. For 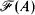, it is natural to identify such a basis with a collection of partial types approximating a certain type.

In this paper, we extend the theory of forking and deal with FN bases (hence ideals in particular). By proving a few results here, we hope to indicate that this extension could become as fruitful as Keisler's theory and hence provide an alternative. Our approach here is more algebraic and less analytic. Unlike [5], methods from nonstandard analysis are not used.

There are two fundamental reasons why the classical theory should be generalized. One is to extend our investigation to unstable theories. (There are no stability assumptions on the theory in the present work.) The other is to study different and more flexible ways of collecting formulas, such as FN basis.

After fixing our notation in §1, we first deal with ideals in §2. We study certain nice extensions. Specifically, we prove the existence of flat nonforking ideals. We then provide a normalization theorem for ideals.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 56 , Issue 3 , September 1991 , pp. 813 - 822
Copyright
Copyright © Association for Symbolic Logic 1991

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Chang, C. C. and Keisler, H. J., Model theory, North-Holland, Amsterdam, 1977.Google Scholar
[2] Drewnowski, L., Topological rings of sets, continuous set functions, integration. I, Bulletin de l'Académie Polonaise des Sciences, Série des Sciences Mathématiques, Astronomiques et Physiques, vol. 20 (1972), pp. 269–276.Google Scholar
[3] Fremlin, D. H., Measure algebras, Handbook of Boolean algebra. Vol. III (Monk, J. D. and Bonnet, R., editors), North-Holland, Amsterdam, 1989, pp. 877–980.Google Scholar
[4] Harnik, V. and Harrington, L., Fundamentals of forking, Annals of Pure and Applied Logic, vol. 26(1984), pp. 245–286.CrossRefGoogle Scholar
[5] Keisler, H. J., Measures and forking, Annals of Pure and Applied Logic, vol. 34 (1987), pp. 119–169.CrossRefGoogle Scholar
[6] Keisler, H. J., Choosing elements in a saturated model, Classification theory (Baldwin, J. T., editor), Lecture Notes in Mathematics, vol. 1292, Springer-Verlag, Berlin, 1987, pp. 165–181.CrossRefGoogle Scholar
[7] Ng, S.A., A nonstandard approach to the theory of forking, Ph.D. Thesis, University of Wisconsin, Madison, Wisconsin, 1988.Google Scholar
[8] Pillay, A., An introduction to stability theory, Clarendon Press, Oxford, 1983.Google Scholar
[9] Pillay, A., Forking, normalization and canonical bases, Annals of Pure and Applied Logic, vol. 32 (1986), pp. 61–81.CrossRefGoogle Scholar
[10] Shelah, S., Classification theory, North-Holland, Amsterdam, 1978.Google Scholar