Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-25T19:04:14.820Z Has data issue: false hasContentIssue false
  • English
  • Français

The independence of a weak axiom of choice1

Published online by Cambridge University Press:  12 March 2014

Elliott Mendelson
Get access Rights & Permissions [Opens in a new window]

Extract

1. The purpose of this paper is to show that, if the axioms of a system G of set theory are consistent, then it is impossible to prove from them the following weak form of the axiom of choice: (H1) For every denumerable set x of disjoint two-element sets, there is a set y, called a choice set for x, which contains exactly one element in common with each element of x. Among the axioms of the system G, we take, with minor modifications, Axioms A, B, C of Gödel [6]. Clearly, the independence of H1 implies that of all stronger propositions, including the general axiom of choice and the generalized continuum hypothesis.

The proof depends upon ideas of Fraenkel and Mostowski, and proceeds in the following manner. Let a be a denumerable set of objects Δ0, Δ1, Δ2, …, the exact nature of which will be specified later. Let μj = {Δ2j, Δ2j+1} for each j, c = {μ0, μ1, μ2, …}, and b = [the sum set of a]. By transfinite induction, construct the class Vc which is the closure of b under the power-set operation. For each j, it is possible to define a 1–1 mapping 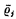of Vc onto itself with the following properties. The mapping preserves the ε-relation, or, more precisely, .

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 21 , Issue 4 , December 1956 , pp. 350 - 366
Copyright
Copyright © Association for Symbolic Logic 1956

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

1

From a thesis in partial fulfillment of the requirements for the degree of Ph. D. in the Department of Mathematics of Cornell University, written while the author was a National Science Foundation Fellow in 1954–1955. The author would like to thank Professor J. Barkley Rosser for many helpful comments on the material of this paper.

References

BIBLIOGRAPHY

[1]Bernays, P., A system of axiomatic set theory, Part VII, this Journal, vol. 19 (1954), pp. 81–96.Google Scholar
[2]Doss, R., Note on two theorems of Mostowski, this Journal, vol. 10 (1945), pp. 13–15.Google Scholar
[3]Fraenkel, A., Der Begriff “definit” und die Unabhängigheit des Auswahlaxioms, Sitzungsberichte der Preussischen Akademie der Wissenschaften, Physikalisch-mathematische Klasse, 1922, pp. 253–257.Google Scholar
[4]Fraenkel, A., Sur l'axiome du choix, L'Enseignement mathématique, vol. 34 (1935), pp. 32–51.Google Scholar
[5]Fraenkel, A.Über eine Abgeschwächte Fassung des Auswahlaxioms, this Journal, vol. 2 (1937), pp. 1–25.Google Scholar
[6]Gödel, K., The consistency of the axiom of choice and of the generalized continuum hypothesis with the axioms of set theory, second printing, Princeton (Princeton University Press) 1951.Google Scholar
[7]Lindenbaum, A. and Mostowski, A., Über die Unabhängigkeit des Auswahlaxioms und einiger seiner Folgerungen, Comptes rendus des séances de la Société des Sciences et des Lettres de Varsovie, Classe III, vol. 15 (1938), pp. 27–32.Google Scholar
[8]Mostowski, A., Über die Unabhängigkeit des Wohlordnungssatzes vom Ordnungsprinzip, Fundamenta mathematicae, vol. 31 (1939), pp. 201–252.CrossRefGoogle Scholar
[9]Mostowski, A., On the principle of dependent choices, Fundamenta mathematicae, vol. 35 (1948), pp. 127–130.CrossRefGoogle Scholar
[10]Mostowski, A., Axiom of choice for finite sets, Fundamenta mathematicae, vol. 32 (1945), pp. 137–168.CrossRefGoogle Scholar
[11]Shepherdson, J. C., Inner models for set theory, Part I, this Journal, vol. 16 (1951), pp. 161–190.Google Scholar
[12]Sierpinski, W., L'hypothese généralisée du continu et l'axiome du choix, Fundamenta mathematicae, vol. 34 (1947), pp. 1–5.CrossRefGoogle Scholar
[13]Specker, E., Verallgemeinerte Kontinuumhypothese und Auswahlaxiom, Archiv der Mathematik, vol. 5 (1954), pp. 332–337.CrossRefGoogle Scholar