Article contents
Indifferent sets for genericity
Published online by Cambridge University Press: 12 March 2014
Abstract
This paper investigates indifferent sets for comeager classes in Cantor space focusing of the class of all 1-generic sets and the class of all weakly 1-generic sets. Jockusch and Posner showed that there exist 1-generic sets that have indifferent sets [10]. Figueira, Miller and Nies have studied indifferent sets for randomness and other notions [7]. We show that any comeager class in Cantor space contains a comeager class with a universal indifferent set. A forcing construction is used to show that any 1-generic set, or weakly 1-generic set, has an indifferent set. Such an indifferent set can by computed by any set in 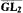 which bounds the (weakly) 1-generic. We show by approximation arguments that some, but not all,
which bounds the (weakly) 1-generic. We show by approximation arguments that some, but not all,  1-generic sets can compute an indifferent set for themselves. We show that all
1-generic sets can compute an indifferent set for themselves. We show that all  weakly 1-generic sets can compute an indifferent set for themselves. Additional results on indifferent sets, including one of Miller, and two of Fitzgerald, are presented.
weakly 1-generic sets can compute an indifferent set for themselves. Additional results on indifferent sets, including one of Miller, and two of Fitzgerald, are presented.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2013
References
REFERENCES
- 4
- Cited by

