Article contents
INITIAL SELF-EMBEDDINGS OF MODELS OF SET THEORY
Published online by Cambridge University Press: 13 August 2021
Abstract
By a classical theorem of Harvey Friedman (1973), every countable nonstandard model  $\mathcal {M}$ of a sufficiently strong fragment of ZF has a proper rank-initial self-embedding j, i.e., j is a self-embedding of
$\mathcal {M}$ of a sufficiently strong fragment of ZF has a proper rank-initial self-embedding j, i.e., j is a self-embedding of  $\mathcal {M}$ such that
$\mathcal {M}$ such that  $j[\mathcal {M}]\subsetneq \mathcal {M}$, and the ordinal rank of each member of
$j[\mathcal {M}]\subsetneq \mathcal {M}$, and the ordinal rank of each member of 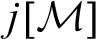 $j[\mathcal {M}]$ is less than the ordinal rank of each element of
$j[\mathcal {M}]$ is less than the ordinal rank of each element of  $\mathcal {M}\setminus j[\mathcal {M}]$. Here, we investigate the larger family of proper initial-embeddings j of models
$\mathcal {M}\setminus j[\mathcal {M}]$. Here, we investigate the larger family of proper initial-embeddings j of models  $\mathcal {M}$ of fragments of set theory, where the image of j is a transitive submodel of
$\mathcal {M}$ of fragments of set theory, where the image of j is a transitive submodel of  $\mathcal {M}$. Our results include the following three theorems. In what follows,
$\mathcal {M}$. Our results include the following three theorems. In what follows,  $\mathrm {ZF}^-$ is
$\mathrm {ZF}^-$ is  $\mathrm {ZF}$ without the power set axiom;
$\mathrm {ZF}$ without the power set axiom; 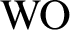 $\mathrm {WO}$ is the axiom stating that every set can be well-ordered;
$\mathrm {WO}$ is the axiom stating that every set can be well-ordered; 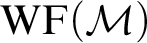 $\mathrm {WF}(\mathcal {M})$ is the well-founded part of
$\mathrm {WF}(\mathcal {M})$ is the well-founded part of  $\mathcal {M}$; and
$\mathcal {M}$; and 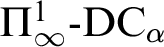 $\Pi ^1_\infty \text{-}\mathrm {DC}_\alpha $ is the full scheme of dependent choice of length
$\Pi ^1_\infty \text{-}\mathrm {DC}_\alpha $ is the full scheme of dependent choice of length 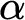 $\alpha $.
$\alpha $.
Theorem A.
There is an  $\omega $-standard countable nonstandard model
$\omega $-standard countable nonstandard model  $\mathcal {M}$ of
$\mathcal {M}$ of 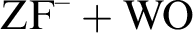 $\mathrm {ZF}^-+\mathrm {WO}$ that carries no initial self-embedding
$\mathrm {ZF}^-+\mathrm {WO}$ that carries no initial self-embedding 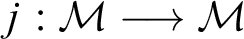 $j:\mathcal {M} \longrightarrow \mathcal {M}$ other than the identity embedding.
$j:\mathcal {M} \longrightarrow \mathcal {M}$ other than the identity embedding.
Theorem B.
Every countable  $\omega $-nonstandard model
$\omega $-nonstandard model  $\mathcal {M}$ of
$\mathcal {M}$ of  $\ \mathrm {ZF}$ is isomorphic to a transitive submodel of the hereditarily countable sets of its own constructible universe
$\ \mathrm {ZF}$ is isomorphic to a transitive submodel of the hereditarily countable sets of its own constructible universe 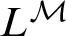 $L^{\mathcal {M}}$.
$L^{\mathcal {M}}$.
Theorem C.
The following three conditions are equivalent for a countable nonstandard model  $\mathcal {M}$ of
$\mathcal {M}$ of 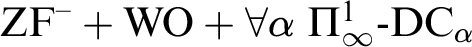 $\mathrm {ZF}^{-}+\mathrm {WO}+\forall \alpha \ \Pi ^1_\infty \text{-}\mathrm {DC}_\alpha $.
$\mathrm {ZF}^{-}+\mathrm {WO}+\forall \alpha \ \Pi ^1_\infty \text{-}\mathrm {DC}_\alpha $.
(I) There is a cardinal in
 $\mathcal {M}$ that is a strict upper bound for the cardinality of each member of
$\mathcal {M}$ that is a strict upper bound for the cardinality of each member of 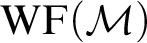 $\mathrm {WF}(\mathcal {M})$.
$\mathrm {WF}(\mathcal {M})$.(II)
 $\mathrm {WF}(\mathcal {M})$ satisfies the powerset axiom.
$\mathrm {WF}(\mathcal {M})$ satisfies the powerset axiom.(III) For all
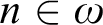 $n \in \omega $ and for all
$n \in \omega $ and for all 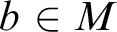 $b \in M$, there exists a proper initial self-embedding
$b \in M$, there exists a proper initial self-embedding 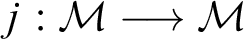 $j: \mathcal {M} \longrightarrow \mathcal {M}$ such that
$j: \mathcal {M} \longrightarrow \mathcal {M}$ such that 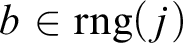 $b \in \mathrm {rng}(j)$ and
$b \in \mathrm {rng}(j)$ and 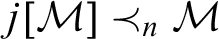 $j[\mathcal {M}] \prec _n \mathcal {M}$.
$j[\mathcal {M}] \prec _n \mathcal {M}$.
MSC classification
Information
- Type
- Article
- Information
- Copyright
- © Association for Symbolic Logic 2021
References
- 1
- Cited by


