Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-25T06:31:35.789Z Has data issue: false hasContentIssue false
  • English
  • Français

Interpolation theorems, lower bounds for proof systems, and independence results for bounded arithmetic

Published online by Cambridge University Press:  12 March 2014

Jan Krajíček
Jan Krajíček*
Affiliation:
Mathematical Institute of the Academy of Sciences, Žitná 25, Praha 1, 115 67, Czech Republic, E-mail: krajicek@mbox.cesnet.cz
Get access Rights & Permissions [Opens in a new window]

Abstract

A proof of the (propositional) Craig interpolation theorem for cut-free sequent calculus yields that a sequent with a cut-free proof (or with a proof with cut-formulas of restricted form; in particular, with only analytic cuts) with k inferences has an interpolant whose circuit-size is at most k. We give a new proof of the interpolation theorem based on a communication complexity approach which allows a similar estimate for a larger class of proofs. We derive from it several corollaries:

  1. (1) Feasible interpolation theorems for the following proof systems:

    1. (a) resolution

    2. (b) a subsystem of LK corresponding to the bounded arithmetic theory 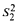(α)

    3. (c) linear equational calculus

    4. (d) cutting planes.

  2. (2) New proofs of the exponential lower bounds (for new formulas)

    1. (a) for resolution ([15])

    2. (b) for the cutting planes proof system with coefficients written in unary ([4]).

  3. (3) An alternative proof of the independence result of [43] concerning the provability of circuit-size lower bounds in the bounded arithmetic theory 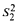(α).

In the other direction we show that a depth 2 subsystem of LK does not admit feasible monotone interpolation theorem (the so called Lyndon theorem), and that a feasible monotone interpolation theorem for the depth 1 subsystem of LK would yield new exponential lower bounds for resolution proofs of the weak pigeonhole principle.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 62 , Issue 2 , June 1997 , pp. 457 - 486
Copyright
Copyright © Association for Symbolic Logic 1997

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Alon, N. and Boppana, R., The monotone circuit complexity of Boolean functions, Combinatorica, vol. 7 (1987), no. 1, pp. 1–22.CrossRefGoogle Scholar
[2]Andreev, A. E., On a method for obtaining lower bounds for the complexity of individual monotone functions, Doklady ANSSSR, vol. 282 (1985), no. 5, pp. 1033–1037, in Russian.Google Scholar
[3]Beth, E. W., The foundations of mathematics, North-Holland, Amsterdam, 1959.Google Scholar
[4]Bonet, M. L., Pitassi, T., and Raz, R., Lower bounds for cutting planes proofs with small coefficients, preprint, 1994.CrossRefGoogle Scholar
[5]Buss, S. R., Bounded arithmetic, Bibliopolis, Naples, 1986.Google Scholar
[6]Buss, S. R. and Turán, G., Resolution proofs of generalized pigeonhole principles, Theoretical Computer Science, vol. 62 (1988), pp. 311–317.CrossRefGoogle Scholar
[7]Chiari, M. and Krajíček, J., Witnessing functions in bounded arithmetic and search problems, submitted, 1994.Google Scholar
[8]Cook, S. A., Feasibly constructive proofs and the prepositional calculus, Proceedings of the 7th Annual ACM Symposium on Theory of Computing, ACM Press, 1975, pp. 83–97.Google Scholar
[9]Cook, S. A. and Reckhow, A. R., The relative efficiency of prepositional proof systems, this Journal, vol. 44 (1979), no. 1, pp. 36–50.Google Scholar
[10]Cook, W., Coullard, C. R., and Turán, G., On the complexity of cutting plane proofs, Discrete Applied Mathematics, vol. 18 (1987), pp. 25–38.CrossRefGoogle Scholar
[11]Craig, W., Linear reasoning: A new form of the Herbrand-Gentzen theorem, this Journal, vol. 22 (1957), no. 3, pp. 250–268.Google Scholar
[12]Craig, W., Three uses of the Herbrand-Gentzen theorem in relating model theory and proof theory, this Journal, vol. 22 (1957), no. 3, pp. 269–285.Google Scholar
[13]Friedman, H., The complexity of explicit definitions, Advances in Mathematics, vol. 20 (1976), pp. 18–29.CrossRefGoogle Scholar
[14]Gurevich, Y., Towards logic tailored for computational complexity, Proceedings of Logic Colloquium 1983 (Berlin), Springer Lecture Notes in Mathematics, no. 1104, Springer-Verlag, 1984, pp. 175–216.Google Scholar
[15]Haken, A., The intractability of resolution, Theoretical Computer Science, vol. 39 (1985), pp. 297–308.CrossRefGoogle Scholar
[16]Karchmer, M. and Wigderson, A., Monotone circuits for connectivity require super-logarithmic depth, Proceedings of the 20th Annual ACM Symposium on Theory of Computing, ACM Press, 1988, pp. 539–550.Google Scholar
[17]Krajíček, J., Exponentiation and second-order bounded arithmetic, Annals of Pure and Applied Logic, vol. 48 (1989), pp. 261–276.CrossRefGoogle Scholar
[18]Krajíček, J., No counter-example interpretation and interactive computation, Logic from Computer Science, Proceedings of a workshop held November 13–17, 1989, in Berkeley, Mathematical Sciences Research Institute Publication (Berlin) (Moschovakis, Y. N., editor), no. 21, Springer-Verlag, 1992, pp. 287–293.Google Scholar
[19]Krajíček, J., Lower bounds to the size of constant-depth prepositional proofs, this Journal, vol. 59 (1994), no. 1, pp. 73–86.Google Scholar
[20]Krajíček, J., Bounded arithmetic, prepositional logic and complexity theory, Cambridge University Press, 1995.CrossRefGoogle Scholar
[21]Krajíček, J., On Frege and extended Frege proof systems, Feasible Mathematics II (Clote, P. and Remmel, J., editors), Birkhäuser, 1995, pp. 284–319.CrossRefGoogle Scholar
[22]Krajíček, J. and Pudlák, P., Prepositional proof systems, the consistency of first order theories and the complexity of computations, this Journal, vol. 54 (1989), no. 3, pp. 1063–1079.Google Scholar
[23]Krajíček, J., Prepositional provability in models of weak arithmetic, Computer Science Logic (Boerger, E., Kleine-Bunning, H., and Richter, M. M., editors), Lecture Notes in Computer Science, no. 440, Springer-Verlag, Berlin, 1990, Kaiserlautern, 10 1989, pp. 193–210.Google Scholar
[24]Krajíček, J., Quantified prepositional calculi and fragments of bounded arithmetic, Zeitschrift für Mathematikal Logik und Grundlagen der Mathematik, vol. 36 (1990), pp. 29–46.CrossRefGoogle Scholar
[25]Krajíček, J., Some consequences of cryptographical conjectures for and EF, Proceedings of the meeting Logic and Computational Complexity (Leivant, D., editor), 1995, Indianapolis, 10 1994, to appear.Google Scholar
[26]Krajíček, J. and Takeuti, G., On bounded -polynomial induction, Feasible mathematics (Buss, S. R. and Scott, P. J., editors), Birkhäuser, 1990, pp. 259–280.CrossRefGoogle Scholar
[27]Krajíček, J., On induction-free provability, Annals of Mathematics and Artificial Intelligence, vol. 6 (1992), pp. 107–126.CrossRefGoogle Scholar
[28]Kreisel, G., Technical report nb. 3, Applied Mathematics and Statistics Labs, Stanford University, unpublished, 1961.Google Scholar
[29]Luby, M., Pseudo-randomness and applications, International Computer Science Institute, Berkeley, lecture notes, 1993.Google Scholar
[30]Mundici, D., A lower bound for the complexity of Craig's interpolants in sentential logic, Archiv für Mathematikal Logik, vol. 23 (1983), pp. 27–36.CrossRefGoogle Scholar
[31]Mundici, D., NP and Craig's interpolation theorem, Proceedings of Logic Colloquium 1982, North-Holland, 1984, pp. 345–358.Google Scholar
[32]Mundici, D., Tautologies with a unique Craig interpolant, uniform vs. non-uniform complexity, Annals of Pure and Applied Logic, vol. 27 (1984), pp. 265–273.CrossRefGoogle Scholar
[33]Papadimitriou, A., Computational complexity, Addison-Wesley, 1994.Google Scholar
[34]Parikh, R., Existence and feasibility in arithmetic, this Journal, vol. 36 (1971), pp. 494–508.Google Scholar
[35]Paris, J. and Wilkie, A. J., Counting problems in bounded arithmetic, Methods in Mathematical Logic, Springer Lecture Notes in Mathematics, no. 1130, Springer-Verlag, Berlin, 1985, pp. 317–340.CrossRefGoogle Scholar
[36]Paris, J., On the scheme of induction for bounded arithmetic formulas, Annals of Pure and Applied Logic, vol. 35 (1987), pp. 261–302.Google Scholar
[37]Paris, J. B., Wilkie, A. J., and Woods, A. R., Provability of the pigeonhole principle and the existence of infinitely many primes, this Journal, vol. 53 (1988), pp. 1235–1244.Google Scholar
[38]Pratt, V. R., Every prime has a succinct certificate, SIAM Journal of Computing, vol. 4 (1975), pp. 214–220.CrossRefGoogle Scholar
[39]Razborov, A. A., Lower bounds on the monotone complexity of some Boolean functions, Soviet Mathem. Doklady, vol. 31 (1985), pp. 354–357.Google Scholar
[40]Razborov, A. A., An equivalence between second order bounded domain bounded arithmetic and first order bounded arithmetic, Arithmetic, Proof Theory and Computational Complexity (Clote, P. and Krajíček, J., editors), Oxford University Press, 1993, pp. 247–277.CrossRefGoogle Scholar
[41]Razborov, A. A., On provably disjoint NP-pairs, preprint, 1994.CrossRefGoogle Scholar
[42]Razborov, A. A., Bounded arithmetic and lower bounds in Boolean complexity, Feasible mathematics II (Clote, P. and Remmel, J., editors), Birkhäuser, 1995, pp. 344–386.CrossRefGoogle Scholar
[43]Razborov, A. A., Unprovability of lower bounds on the circuit size in certain fragments of bounded arithmetic, Izvestiya of the R. A. N., vol. 59 (1995), no. 1, pp. 201–224.Google Scholar
[44]Razborov, A.A. and Rudich, S., Natural proofs, Proceedings of the 26th Annual ACM Symposium on Theory of Computing, ACM Press, 1994, pp. 204–213.Google Scholar
[45]Takeuti, G., Proof theory, North-Holland, 1975.Google Scholar
[46]Takeuti, G., RSUV isomorphism, Arithmetic, Proof Theory and Computational Complexity (Clote, P. and Krajíček, J., editors), Oxford University Press, 1993, pp. 364–386.CrossRefGoogle Scholar