No CrossRef data available.
Article contents
Large resplendent models generated by indiscernibles
Published online by Cambridge University Press: 12 March 2014
Extract
The motivation for the results presented here comes from the following two known theorems which concern countable, recursively saturated models of Peano arithmetic.
(1) if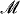 
is a countable, recursively saturated model of PA, then for each infinite cardinal κ there is a resplendent 
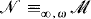which has cardinality κ. (See Theorem 10 of [1].)
(2) if 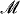is a countable, recursively saturated model of PA, then 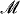is generated by a set of indiscernibles. (See [4].)
It will be shown here that (1) and (2) can be amalgamated into a common generalization.
(3) if 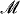is a countable, recursively saturated model of PA, then for each infinite cardinal κ there is a resplendent 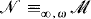which has cardinality κ and which is generated by a set of indiscernibles.
By way of contrast we will also get recursively saturated models of PA which fail to be resplendent and yet are generated by indiscernibles.
(4) if 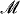is a countable, recursively saturated model of PA, then for each uncountable cardinal κ there is a κ-like recursively saturated 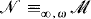generated by a set of indiscernibles.
None of (1), (2) or (3) is stated in its most general form. We will make some comments concerning their generalizations. From now on let us fix a finite language L; all structures considered are infinite L-structures unless otherwise indicated.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1989
References
REFERENCES
[1]
Buechler, S., Expansion of models of ω-stable theories, this Journal, vol. 49 (1984), pp. 470–477.Google Scholar
[2]
Schlipf, J. S., A guide to the identification of admissible sets above structures, Annals of Mathematical Logic, vol. 12 (1977), pp. 151–192.CrossRefGoogle Scholar
[3]
Schmerl, J. H., Recursively saturated, rather classless models of Peano arithmetic, Logic year 1979–80, Lecture Notes in Mathematics, vol. 859, Springer-Verlag, Berlin, 1981, pp. 262–282.CrossRefGoogle Scholar
[4]
Schmerl, J. H., Recursively saturated models generated by indiscernibles, Notre Dame Journal of Formal Logic, vol. 26 (1985), pp. 99–105.CrossRefGoogle Scholar