Article contents
A MARRIAGE OF BROUWER’S INTUITIONISM AND HILBERT’S FINITISM I: ARITHMETIC
Published online by Cambridge University Press: 07 January 2021
Abstract
We investigate which part of Brouwer’s Intuitionistic Mathematics is finitistically justifiable or guaranteed in Hilbert’s Finitism, in the same way as similar investigations on Classical Mathematics (i.e., which part is equiconsistent with 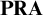 $\textbf {PRA}$ or consistent provably in
$\textbf {PRA}$ or consistent provably in 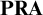 $\textbf {PRA}$) already done quite extensively in proof theory and reverse mathematics. While we already knew a contrast from the classical situation concerning the continuity principle, more contrasts turn out: we show that several principles are finitistically justifiable or guaranteed which are classically not. Among them are: (i) fan theorem for decidable fans but arbitrary bars; (ii) continuity principle and the axiom of choice both for arbitrary formulae; and (iii)
$\textbf {PRA}$) already done quite extensively in proof theory and reverse mathematics. While we already knew a contrast from the classical situation concerning the continuity principle, more contrasts turn out: we show that several principles are finitistically justifiable or guaranteed which are classically not. Among them are: (i) fan theorem for decidable fans but arbitrary bars; (ii) continuity principle and the axiom of choice both for arbitrary formulae; and (iii) 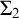 $\Sigma _2$ induction and dependent choice. We also show that Markov’s principle MP does not change this situation; that neither does lesser limited principle of omniscience LLPO (except the choice along functions); but that limited principle of omniscience LPO makes the situation completely classical.
$\Sigma _2$ induction and dependent choice. We also show that Markov’s principle MP does not change this situation; that neither does lesser limited principle of omniscience LLPO (except the choice along functions); but that limited principle of omniscience LPO makes the situation completely classical.
Keywords
Information
- Type
- Article
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press on behalf of The Association for Symbolic Logic
References
- 5
- Cited by


