Martin's axiom in the model theory of L_A

Extract

Working in ZFC + Martin's Axiom we develop a generalization of the Barwise Compactness Theorem which holds in languages of cardinality less than 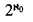. Next, using this compactness theorem, an omitting types theorem for fewer than 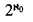types is proved. Finally, in ZFC, we prove that this compactness result implies Martin's Axiom (the Equivalence Theorem). Our compactness theorem applies to a new class of theories—ccΣ-theories—which generalize the countable Σ-theories of Barwise's theorem. The Omitting Types Theorem and the Equivalence Theorem serve as examples illustrating the use of ccΣ-theories.

Assume = (A, ε) or 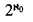= (A, ε R₁,…,R_m) where 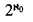is admissible. L(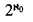) is the first-order language with constants for elements of A and relation symbols for relations in 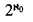. L_A is A ⋂ L_∞ω where the L of L_∞ω is any language in A. A theory T in L_A is consistent if there is no derivation in A of a contradiction from T. 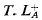is L_A with new constants c_a for each a and A. The basic terms of 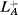consist of the constants of 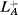and the terms f(c_a1,…,c_am) built directly from constants using functions f of 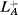. The symbol t is used for basic terms. A theory T in L_A is Σ if it is defined by a 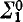formula of L(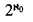). The formula φ^⌝ is a logical equivalent of ¬φ defined by: (1) φ^⌝ = ¬φ if φ is atomic; (2) (¬φ)^⌝ = φ (3) (⋁_φ∈Φ φ)^⌝ = ⋀_φ∈Φ φ^⌝; (4) (⋀_φ∈Φ φ) ⋁_φ∈Φ φ^⌝; (5) (∃χφ(x))^⌝ ∀χφ^⌝(x); ∀χφ(x))^⌝ = ∃χφ^⌝(x).