No CrossRef data available.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
The purpose of this paper is to introduce the notion of “omitting models” and to derive a very natural theorem concerning it (Theorem 1). A corollary of this theorem is the remarkable theorem of Vaught [3] which states that a countable complete theory cannot have precisely two nonisomorphic countable models. In fact, we show that our theorem implies Rosenstein's theorem [2] which, in turn, implies Vaught's theorem.
T stands for a countable complete theory whose (countable) language is denoted by L. Following [1], a countably homogeneous model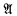 
of T is a countable model of T with the property that, for any two n-tuples a1, …, an and b1,…,bn of the universe of 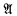whose types are the same, there is an automorphism of 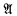which maps ai, on bi, for i = 1, …, n [1, p. 129 and Proposition 3.2.9, p. 131]. “Homogeneous model” always means “countably homogeneous model.” “Type of T” always stands for “n-type of T” where n ≥ s 0, i.e., for the type of some n-tuple of individuals of the universe of some model of T. We often use that two homogeneous models which realize the same types are isomorphic [1, Proposition 3.2.9, p. 131].
It is well known that every type of T is realized by at least one countable model of T. The main definition of this paper is:
Definition 1. A set of countable models of T is omissible or “may be omitted” if every type of T is realized by at least one countable model of T which is not isomorphic to a model in the set.
The main theorem of the paper is:
Theorem 1. If a countable complete theory is not ω-categorical, every finite set of its homogeneous models may be omitted.
The theorem is proved in §1 and in §2 it is shown how Vaught's and Rosenstein's theorems follow from it. §3 discusses some general aspects of omitting models.