No CrossRef data available.
Article contents
On the consistency of self-referential systems
Published online by Cambridge University Press: 12 March 2014
Extract
In [2], Hiller and myself have introduced what we have called self-referential systems. Under this name we categorize models of a theory 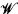 whose predicates refer to objects which are themselves predicates of the language—this particular interpretation being considered as the intended one.
whose predicates refer to objects which are themselves predicates of the language—this particular interpretation being considered as the intended one.
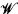 is formulated in a language whose variables are typed: as types become “larger”, the corresponding objects become “smaller”; for this reason, we have suggestively chosen types to be negative integers: 0, −1, −2, and so on.
is formulated in a language whose variables are typed: as types become “larger”, the corresponding objects become “smaller”; for this reason, we have suggestively chosen types to be negative integers: 0, −1, −2, and so on.
The consistency of 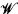 has been questioned several times, and it has also been referred to as an “intriguing possibility”. Under such motivation, we are most willing to present a consistency proof for
has been questioned several times, and it has also been referred to as an “intriguing possibility”. Under such motivation, we are most willing to present a consistency proof for 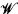 , and we do it relative to the theory ZFC + “there exists a Ramsey cardinal”. The only use of the Ramsey cardinal is to justify the existence of 0#, and the existence of 0# is known to be weaker than the existence of a Ramsey cardinal. For this reason, we note that the minimum we need for our proof is the existence of the set 0#.
, and we do it relative to the theory ZFC + “there exists a Ramsey cardinal”. The only use of the Ramsey cardinal is to justify the existence of 0#, and the existence of 0# is known to be weaker than the existence of a Ramsey cardinal. For this reason, we note that the minimum we need for our proof is the existence of the set 0#.
To begin with, we prove that the consistency of  implies the existence of intended models for
implies the existence of intended models for  : these are models in which every element is definable by a one-free-variable formula of the language. In intended models we also show that the axiom
: these are models in which every element is definable by a one-free-variable formula of the language. In intended models we also show that the axiom 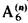 holds, and, besides, these models possess
holds, and, besides, these models possess  -indescribable cardinals. These two results imply the relative consistency of
-indescribable cardinals. These two results imply the relative consistency of 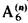 with respect to
with respect to 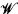 and, moreover, that any proof of the consistency of
and, moreover, that any proof of the consistency of 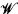 cannot be carried out without the use of some large cardinal axiom: such an axiom which, when added to ZFC, produces a theory equiconsistent to
cannot be carried out without the use of some large cardinal axiom: such an axiom which, when added to ZFC, produces a theory equiconsistent to 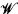 —if it exists—remains unknown. At the end of this article we finally exhibit the consistency proof of
—if it exists—remains unknown. At the end of this article we finally exhibit the consistency proof of 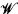 , our main result.
, our main result.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1987

