No CrossRef data available.
Article contents
Preservation theorem and relativization theorem for cofinal extensions1
Published online by Cambridge University Press: 12 March 2014
Extract
One of the typical preservation theorems in a first order classical predicate logic with equality L is the following theorem due to J. Łoś [4] and A. Tarski [9] (also cf. [1, p. 139]).
Theorem A (Łoś-Tarski). For any sentences A and B in L, the following two conditions (i) and (ii) are equivalent.
(i) Every extension of any model of A is a model of B.
(ii) The two sentences A ⊃ C and C ⊃ B are provable in L for some existential sentence C in L.
In [2], S. Feferman obtained a similar preservation theorem for outer extensions. In the following, we assume that L has a fixed binary predicate symbol <. Then Σ-formulas are formulas in L which are constructed from atomic formulas and their negations by applying ∧ (conjunctions), ∨ (disjunctions), ∀x < y (bounded universal quantifications), and ∃ (existential quantifications). An extension 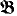 of an L-structure
of an L-structure 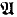 is said to be an outer extension of
is said to be an outer extension of 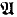 if
if 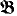 ⊨ a < b and b ϵ ∣
⊨ a < b and b ϵ ∣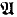 ∣ imply a ϵ ∣
∣ imply a ϵ ∣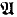 ∣ for any elements a, b in ∣
∣ for any elements a, b in ∣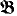 ∣.
∣.
Theorem B (Feferman). For any sentences A and B in L, the following two conditions (i) and (ii) are equivalent.
(i) Every outer extension of any model of A is a model of B.
(ii) The two sentences A ⊃ C and C ⊃ B are provable in L for some Σ-sentence C in L.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1986
Footnotes
This paper was presented at the special session on proof theory at the January 1983 Annual Meeting of the American Mathematical Society in Denver, Colorado; unfortunately its appearance in print has been delayed until now.

