Article contents
Real functions on the family of all well-ordered subsets of a partially ordered set1
Published online by Cambridge University Press: 12 March 2014
Extract
Definition 1 (Kurepa [3, p. 99]). Let E be a partially ordered set. Then σE denotes the set of all bounded well-ordered subsets of E. We consider σE as a partially ordered set with ordering 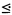 defined as follows: s
defined as follows: s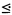 t if and only if s is an initial segment of t.
t if and only if s is an initial segment of t.
Then σE is a tree, i.e., {s ∈ σ E∣ s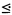 t} is well-ordered for every t ∈ σE. The trees of the form αE were extensively studied by Kurepa in [3]–[10]. For example, in [4], he used σQ and σR to construct various sorts of Aronszajn trees. (Here Q and R denote the rationals and reals, respectively.) While considering monotone mapping between some kind of ordered sets, he came to the following two questions several times:
t} is well-ordered for every t ∈ σE. The trees of the form αE were extensively studied by Kurepa in [3]–[10]. For example, in [4], he used σQ and σR to construct various sorts of Aronszajn trees. (Here Q and R denote the rationals and reals, respectively.) While considering monotone mapping between some kind of ordered sets, he came to the following two questions several times:
P.1. Does there exist a strictly increasing rational function on σQ? (See [4, Problème 2], [5, p. 1033], [6, p. 841], [7, Problem 23.3.3].)
P.2. Let T be a tree in which every chain is countable and every level has cardinality <2ℵ0. Does there exist a strictly increasing real function on T? (See [6, p. 246] and [7].)
It is known today that Problem 2 is independent of the usual axioms of set theory (see [1]). Concerning Problem 1 we have the following.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1983
Footnotes
This paper was written while we were visiting the Hebrew University of Jerusalem in the first part of 1980. We wish to express here our gratitude to the Department of Mathematics for its hospitality.
References
REFERENCES
- 3
- Cited by

