Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
1971.
Model Theory For Infinitary Logic.
Vol. 62,
Issue. ,
p.
193.
Feferman, Solomon
1972.
Conference in Mathematical Logic — London ’70.
Vol. 255,
Issue. ,
p.
63.
Flum, J�rg
1974.
On Horn theories.
Mathematische Zeitschrift,
Vol. 138,
Issue. 3,
p.
205.
Eklof, Paul C.
1975.
Model Theory and Algebra.
Vol. 498,
Issue. ,
p.
91.
Kueker, David W.
1975.
Infinitary Logic: In Memoriam Carol Karp.
Vol. 492,
Issue. ,
p.
17.
1975.
Large Infinitary Languages - Model Theory.
Vol. 83,
Issue. ,
p.
438.
Eklof, Paul C.
1976.
Infinitary model theory of abelian groups.
Israel Journal of Mathematics,
Vol. 25,
Issue. 1-2,
p.
97.
Nadel, Mark
and
Stavi, Jonathan
1978.
𝐿_{∞}_{𝜆}-equivalence, isomorphism and potential isomorphism.
Transactions of the American Mathematical Society,
Vol. 236,
Issue. 0,
p.
51.
Benda, Miroslav
1979.
Modeloids. I.
Transactions of the American Mathematical Society,
Vol. 250,
Issue. 0,
p.
47.
Mekler, Alan H.
1982.
Primitive rings are not definable in l∞∞.
Communications in Algebra,
Vol. 10,
Issue. 15,
p.
1689.
1990.
Model Theory.
Vol. 73,
Issue. ,
p.
623.
Sturm, Holger
2000.
Elementary Classes in Basic Modal Logic.
Studia Logica,
Vol. 64,
Issue. 2,
p.
193.
Väänänen, Jouko
and
Wang, Tong
2013.
An Ehrenfeucht‐Fraïssé game for .
Mathematical Logic Quarterly,
Vol. 59,
Issue. 4-5,
p.
357.
Adámek, J.
and
Rosický^*, J.
2015.
On reflective subcategories of locally presentable categories.
Theory and Applications of Categories,
Vol. 30,
Issue. ,
p.
1306.

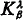 (relations between relational struc tures) and we show their connection with the equivalence of the languages
(relations between relational struc tures) and we show their connection with the equivalence of the languages 