Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-25T06:51:29.877Z Has data issue: false hasContentIssue false
  • English
  • Français

Replacement and collection in intuitionistic set theory

Published online by Cambridge University Press:  12 March 2014

Nicolas D. Goodman
Nicolas D. Goodman*
Affiliation:
Department of Mathematics, State University of New Yorkat Buffalo, Buffalo, New York 14214
Get access Rights & Permissions [Opens in a new window]

Extract

Intuitionistic Zermelo-Fraenkel set theory, which we call ZFI, was introduced by Friedman and Myhill in [3] in 1970. The idea was to have a theory with the same axioms as ordinary classical ZF, but with Heyting's predicate calculus HPC as the underlying logic. Since some classically equivalent statements are intuitionistically inequivalent, however, it was not always obvious which form of a classical axiom to take. For example, the usual formulation of the axiom of foundation had to be replaced with a principle of transfinite induction on the membership relation in order to avoid having excluded middle provable and thus making the logic classical. In [3] the replacement axiom is taken in its most common classical form:

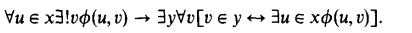
In the presence of the separation axiom,

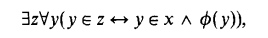
this is equivalent to the axiom

It is this schema Rep that we shall call the replacement axiom.

Friedman and Myhill were able to show in [3] that ZFI has a number of desirable “constructive” properties, including the existence property for both numbers and sets. They were not able to determine, however, whether ZFI is proof-theoretically as strong as ZF. This is still open.

Three years later, in [2], Friedman introduced a theory ZF− which is like ZFI except that the replacement axiom is changed to the classically equivalent collection axiom:

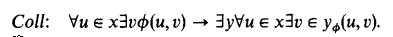
He showed that ZF− is proof-theoretically of the same strength as ZF, and he asked whether ZF− is equivalent to ZFI.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 50 , Issue 2 , June 1985 , pp. 344 - 348
Copyright
Copyright © Association for Symbolic Logic 1985

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Beeson, M., Continuity in intuitionistic set theories, Logic Colloquium '78 (Boffa, M., van Dalen, D. and McAloon, K., editors), North-Holland, Amsterdam, 1979, pp. 1–52.Google Scholar
[2] Friedman, H., The consistency of classical set theory relative to a set theory with intuitionistic logic, this Journal, vol. 38 (1973), pp. 315–319.Google Scholar
[3] Myhill, J., Some properties of intuitionistic Zermelo-Fraenkel set theory, Cambridge summer school in mathematical logic (Mathias, A. R. D. and Rogers, H., editors), Lecture Notes in Mathematics, vol. 337, Springer-Verlag, Berlin, 1973, pp. 206–231.CrossRefGoogle Scholar
[4] Smoryński, C. A., Applications of Kripke models, Metamathematical investigation of intuitionistic arithmetic and analysis (Troelstra, A. S., editor), Lecture Notes in Mathematics, vol. 344, Springer-Verlag, Berlin, 1973, pp. 324–391.CrossRefGoogle Scholar