Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-25T19:29:54.590Z Has data issue: false hasContentIssue false
  • English
  • Français

Saturated ideals

Published online by Cambridge University Press:  12 March 2014

Kenneth Kunen
Kenneth Kunen*
Affiliation:
University of Wisconsin-Madison, Madison, Wisconsin 53706
Get access Rights & Permissions [Opens in a new window]

Extract

In this paper, we give consistency proofs for the existence of a κ-saturated ideal on an inaccessible κ, and for the existence of an ω2-saturated ideal on ω1. We also include an historical survey outlining other known results on saturated ideals.

§1. Forcing. We assume that the reader is familiar with the usual techniques in forcing and Boolean-valued models (see Jech [3] or Rosser [11]), so we shall just specify here some of the less standard notation.

“cBa” abbreviates “complete Boolean algebra”.

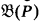If P (= ‹P, <›) is a notion of forcing, is the associated cBa. We write VP for .

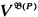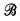Notions like κ-closed, κ-complete, etc. always mean < κ. Thus, P is κ-closed iff every decreasing chain of length less than κ has a lower bound, and a Boolean algebra is κ-complete iff sups of subsets of 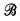of cardinality less than κ always exist.

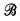If is a cBa, x̌ is the object in 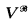representing x in V. In many cases, especially with ordinals, the ̌ is dropped. V̌ is the Boolean-valued class representing V — i.e.,

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 43 , Issue 1 , March 1978 , pp. 65 - 76
Copyright
Copyright © Association for Symbolic Logic 1978

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Baumgartner, J. E., Results and independence proofs in combinatorial set theory, Doctoral Dissertation, University of California, Berkeley, 1970.Google Scholar
[2]Boos, W., Boolean extensions which efface the Mahlo property, this Journal, vol. 39 (1974), pp. 254–268.Google Scholar
[3]Jech, T. J., Lectures in set theory, Lecture Notes in Mathematics, no. 217, Springer-Verlag, Berlin, 1971.Google Scholar
[4]Jech, T. J., Two remarks on elementary embeddings of the universe, Pacific Journal of Mathematics, vol. 39 (1971), pp. 395–400.CrossRefGoogle Scholar
[5]Jensen, R. B., The fine structure of the constructible hierarchy, Annals of Mathematical Logic, vol. 4 (1972), pp. 229–308.CrossRefGoogle Scholar
[6]Kunen, K., Some applications of iterated ultrapowers in set theory, Annals of Mathematical Logic, vol. 1 (1970), pp. 179–227.CrossRefGoogle Scholar
[7]Kunen, K., Elementary embeddings and infinitary combinations, this Journal, vol. 36 (1971), pp. 407–413.Google Scholar
[8]Kunen, K. and Paris, J. B., Boolean extensions and measurable cardinals, Annals of Mathematical Logic, vol. 2 (1971), pp. 359–378.CrossRefGoogle Scholar
[9]Menas, T. K., On strong compactness and supercompactness, Doctoral Dissertation, University of California, Berkeley, 1973.Google Scholar
[10]Prikry, K. L., Changing measurable into accessible cardinals, Dissertationes Mathematicae, Rozprawy Matematyczne, vol. 68 (1970).Google Scholar
[11]Rosser, J. B., Simplified independence proofs, Pure and Applied Mathematics, vol. 31, Academic Press, New York, 1969.Google Scholar
[12]Smith, E. C. Jr. and Tarski, A., Higher degrees of distributivity and completeness in Boolean algebras, Transactions of the American Mathematical Society, vol. 84 (1957), pp. 230–257.CrossRefGoogle Scholar
[13]Solovay, R. M., Real-valued measurable cardinals, Proceedings of Symposia in Pure Mathematics, vol. 13, Part I, 1967 UCLA Summer Institute, American Mathematical Society, Providence, Rhode Island, 1971, pp. 397–428.Google Scholar
[14]Solovay, R. M. and Tennenbaum, S., Iterated Cohen extensions and Souslin's problem, Annals of Mathematics, vol. 94 (1971), pp. 201–245.CrossRefGoogle Scholar
[15]Tarski, A., Ideale in vollstandigen Mengenkörpen II, Fundamenta Mathematicae, vol. 33 (1945), pp. 51–65.CrossRefGoogle Scholar
[16]Ulam, S., Zur Masstheorie in der allgemeinen Mengenlehre, Fundamenta Mathematicae, vol. 16 (1930), pp. 140–150.CrossRefGoogle Scholar