No CrossRef data available.
Article contents
A saturation property of ideals and weakly compact cardinals1
Published online by Cambridge University Press: 12 March 2014
Extract
Suppose κ is a regular uncountable cardinal, λ is a cardinal > 1, and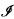 
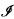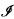is a κ-complete uniform ideal on κ. This paper deals with a saturation property Sat(κ, λ, ) of , which is a weakening of usual λ-saturatedness. Roughly speaking, Sat(κ, λ, 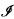) means that 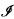can be densely extended to λ-saturated ideals on small fields of subsets of κ. We will show that some consequences of the existence of a λ-saturated ideal on κ follow from weaker ∃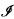: Sat(κ, λ, 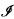), and that ∃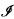: Sat(κ, λ, 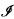) is connected with weak compactness and complete ineffability of κ in much the same way as the existence of a saturated ideal on κ is connected with measurability of κ.
In §2, we define Sat(κ, λ, 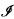), mention a few results that can be proved by straightforward adaptation of known methods, and discuss generic ultrapowers of ZFC−, which will be used repeatedly in the subsequent sections as a main technical tool. A related concept Sat(κ, λ) is also defined and shown to be equivalent to ∃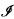: Sat(κ, λ, 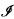) under a certain condition.
In §3, we show that ∃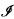: Sat(κ, κ, 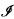) implies that κ is highly Mahlo, improving results in [KT] and [So].
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1986
Footnotes
1
The results in this paper are from the author's Ph.D. thesis [Ta] written under the supervision of Karel Prikry, to whom the author is grateful.
References
REFERENCES
[AHKZ]Abramson, F. G., Harrington, L. A., Kleinberg, E. M. and Zwicker, W. S., Flipping properties: a unifying thread in the theory of large cardinals, Annals of Mathematical Logic, vol. 12 (1977), pp. 25–58.CrossRefGoogle Scholar
[BTW]Baumgartner, J. E., Taylor, A. D. and Wagon, S., Structural properties of ideals, Dissertationes Mathematicae (Rozprawy Matematyczne), vol. 197 (1982).Google Scholar
[Ca]Carlson, T., Extending Lebesgue measure by infinitely many sets, Abstracts of Papers Presented to the American Mathematical Society, vol. 1 (1980), p. 347 (abstract 80T-E27).Google Scholar
[Ka]Kakuda, Y., Saturated ideals in Boolean extensions, Nagoya Mathematical Journal, vol. 48 (1972), pp. 159–168.CrossRefGoogle Scholar
[Kl]Kleinberg, E. M., A combinatorial characterization of normal M-ultrafilters, Advances in Mathematics, vol. 30 (1978), pp. 77–84.CrossRefGoogle Scholar
[KT]Keisler, H. J. and Tarski, A., From accessible to inaccessible cardinals, Fundament a Mathematicae, vol. 53 (1964), pp. 225–308.CrossRefGoogle Scholar
[Ku]Kunen, K., Some applications of iterated ultrapowers in set theory, Annals of Mathematical Logic, vol. 1 (1970), pp. 179–227.CrossRefGoogle Scholar
[Pa]Paris, J. B., Boolean extensions and large cardinals, Ph.D. thesis, Manchester University, Manchester, 1969.Google Scholar
[Pr]Prikry, K., Changing measurable into accessible cardinals, Dissertationes Mathematicae (Rozprawy Matematycze), vol. 68 (1970).Google Scholar
[Si]Silver, J. H., Some applications of model theory in set theory, Annals of Mathematical Logic, vol. 3 (1971), pp. 45–110.CrossRefGoogle Scholar
[So]Solovay, R. M., Real-valued measurable cardinals, Axiomatic set theory, Proceedings of Symposia in Pure Mathematics, vol. 13, part 1, American Mathematical Society, Providence, Rhode Island, 1971, pp. 397–428.CrossRefGoogle Scholar
[ST]Solovay, R. M. and Tennenbaum, S., Iterated Cohen extensions and Souslin's problem, Annals of Mathematics, ser. 2, vol. 94 (1971), pp. 201–245.CrossRefGoogle Scholar
[Ta]Takahashi, J., Partition and saturation properties of ideals, Ph.D. thesis, University of Minnesota, Minneapolis, Minnesota, 1982.Google Scholar
[Tar]Tarski, A., Drei (Überdeckungssätze der allgemeinen Mengenlehre, Fundamenta Mathematicae, vol. 30 (1938), pp. 132–155.CrossRefGoogle Scholar
[Ul]Ulam, S., Zur Masstheorie in der allgemeinen Mengenlehre, Fundamenta Mathematicae, vol. 16 (1930), pp. 140–150.CrossRefGoogle Scholar