Article contents
A simplification of the Bachmann method for generating large countable ordinals
Published online by Cambridge University Press: 12 March 2014
Extract
In [2] Bachmann showed how a hierarchy of normal functions on the countable ordinals Ω can be constructed using certain uncountable ordinals together with appropriate fundamental sequences for limit ordinals, as indexing ordinals for the hierarchy. This method, explained in more detail in §1 below, has been generalized by Pfeiffer [6] and Isles [4] to produce larger hierarchies. Using the hierarchies constructed in this way it is possible, as an immediate consequence of the definitions, to assign ω-sequences 〈αn〉n∈ω to limit ordinals α in an initial segment I of Ω such that 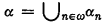 . Indeed this was the motivation for Bachmann's original construction. However the initial segments I constructed in this way are of interest even without the fundamental sequences because they occur naturally as the proof-theoretic ordinals associated with particular formal theories. The fundamental sequences necessary for the Bachmann method are not intrinsically of interest proof-theoretically and only serve to obscure the definitions of the associated initial segments. Feferman and Weyhrauch [9] suggested an alternative definition for a sequence of functions on the countable ordinals. This definition was generalized by Aczel [1] who showed how the new functions corresponded to those in Bachmann's hierarchy. (Independently, Weyhrauch established the same results for an initial segment of the sequence of Bachmann functions, i.e. those functions indexed by α < εΩ+1.)
. Indeed this was the motivation for Bachmann's original construction. However the initial segments I constructed in this way are of interest even without the fundamental sequences because they occur naturally as the proof-theoretic ordinals associated with particular formal theories. The fundamental sequences necessary for the Bachmann method are not intrinsically of interest proof-theoretically and only serve to obscure the definitions of the associated initial segments. Feferman and Weyhrauch [9] suggested an alternative definition for a sequence of functions on the countable ordinals. This definition was generalized by Aczel [1] who showed how the new functions corresponded to those in Bachmann's hierarchy. (Independently, Weyhrauch established the same results for an initial segment of the sequence of Bachmann functions, i.e. those functions indexed by α < εΩ+1.)
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1975
References
BIBLIOGRAPHY
- 21
- Cited by

