No CrossRef data available.
Article contents
Universal sets for pointsets properly on the nth level of the projective hierarchy
Published online by Cambridge University Press: 12 March 2014
Abstract
The Axiom of Projective Determinacy implies the existence of a universal 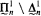 set for every n ≥ 1. Assuming
set for every n ≥ 1. Assuming  there exists a universal
there exists a universal  set. In ZFC there is a universal
set. In ZFC there is a universal 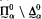 set for every α.
set for every α.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 2013
References
REFERENCES
[1]Barwise, K. J., Gandy, R. O., and Moschovakis, Y. N., The next admissible set, this Journal, vol. 36 (1971), pp. 108–120.Google Scholar
[2]Fremlin, D. H., Consequences of Martin's axiom, Cambridge Tracts in Mathematics, 84, Cambridge University Press, Cambridge, 1984.CrossRefGoogle Scholar
[3]Harrington, Leo, Long projective wellorderings, Annul of Mathematical Logic, vol. 12 (1977), no. 1, pp. 1–24.CrossRefGoogle Scholar
[4]Hjorth, Greg, Universal co-analytic sets, Proceedings of the American Mathematical Society, vol. 124 (1996), no. 12, pp. 3867–3873.CrossRefGoogle Scholar
[6]Kanamori, Akihiro, The higher infinite. Large cardinals in set theory from their beginnings, Perspectives in Mathematical Logic, Springer, 1994.Google Scholar
[7]Kechris, Alexander S., The theory of countable analytical sets, Transactions of the American Mathematical Society, vol. 202 (1975), pp. 259–297.CrossRefGoogle Scholar
[8]Kechris, Alexander S., Classical descriptive set theory, Graduate Texts in Mathematics, 156, Springer, 1995.CrossRefGoogle Scholar
[9]Kunen, Kenneth, Set theory. An introduction to independence proofs, Studies in Logic and the Foundations of Mathematics, vol. 102, North-Holland, 1983, reprint of the 1980 original.Google Scholar
[10]Mansfield, R. and Weitkamp, G., Recursive uspects of descriptive set theory, Oxford University Press, 1985.Google Scholar
[11]Martin, Donald A., Borel determinacy, Annals of Mathematics, Series 2, vol. 102 (1975), no. 2, pp. 363–371.CrossRefGoogle Scholar
[12]Martin, Donald A. and Solovay, R. M., Internal Cohen extensions, Annals of Mathematical Logic, vol. 2 (1970), no. 2, pp. 143–178.CrossRefGoogle Scholar
[13]Miller, Arnold W., Some interesting problems, http://www.math.wisc.edu/~miller/res/problem.pdf.Google Scholar
[15]Rudin, M. E., Martin's axiom, Handbook of mathematical logic (Barwise, Jon, editor), Studies in Logic and the Foundations of Mathematics, vol. 90, North-Holland, 1977, pp. 419–502.CrossRefGoogle Scholar
[17]Steel, J. R., Analytic sets and borel isomorphisms, Fundamenta Mathematica, vol. 108 (1980), no. 2, pp. 83–88.CrossRefGoogle Scholar

