No CrossRef data available.
Article contents
USUBA’S PRINCIPLE 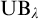 $\mathrm {UB}_\lambda $ CAN FAIL AT SINGULAR CARDINALS
$\mathrm {UB}_\lambda $ CAN FAIL AT SINGULAR CARDINALS
Part of:
Set theory
Published online by Cambridge University Press: 07 September 2023
Abstract
We answer a question of Usuba by showing that the combinatorial principle 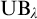 $\mathrm {UB}_\lambda $ can fail at a singular cardinal. Furthermore,
$\mathrm {UB}_\lambda $ can fail at a singular cardinal. Furthermore, 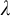 $\lambda $ can be taken to be
$\lambda $ can be taken to be  $\aleph _\omega .$
$\aleph _\omega .$
MSC classification
Information
- Type
- Article
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press on behalf of The Association for Symbolic Logic
References
Eskew, M. and Hayut, Y.,
On the consistency of local and global versions of Chang’s conjecture
.
Transactions of the American Mathematical Society
, vol. 370 (2018), no. 4, pp. 2879–2905. Erratum:
Transactions of the American Mathematical
Society, vol. 374 (2021), no. 1, p. 753.CrossRefGoogle Scholar
Hayut, Y.,
Magidor–Malitz reflection
.
Archive for Mathematical Logic
, vol. 56 (2017), nos. 3–4, pp. 253–272.CrossRefGoogle Scholar
Levinski, J.-P., Magidor, M., and Shelah, S.,
Chang’s conjecture for
 ${\aleph}_{\omega }$
.
Israel Journal of Mathematics
, vol. 69 (1990), no. 2, pp. 161–172.CrossRefGoogle Scholar
${\aleph}_{\omega }$
.
Israel Journal of Mathematics
, vol. 69 (1990), no. 2, pp. 161–172.CrossRefGoogle Scholar
Shelah, S., Non-reflection of the bad set for
 ${\check{I}}_{\theta}[\lambda]$
and pcf. Acta Mathematica Hungarica, vol. 141 (2013), nos. 1–2, pp. 11–35.CrossRefGoogle Scholar
${\check{I}}_{\theta}[\lambda]$
and pcf. Acta Mathematica Hungarica, vol. 141 (2013), nos. 1–2, pp. 11–35.CrossRefGoogle Scholar
Usuba, T.,
New combinatorial principle on singular cardinals and normal ideals
.
Mathematical Logic Quarterly
, vol. 64 (2018), nos. 4–5, pp. 395–408.CrossRefGoogle Scholar


