Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Pour-El, Marian Boykan
and
Richards, Ian
1984.
𝐿^{𝑝}-computability in recursive analysis.
Proceedings of the American Mathematical Society,
Vol. 92,
Issue. 1,
p.
93.
Cantini, Andrea
1985.
On Weak Theories of Sets and Classes which are Based on Strict ∏‐REFLECTION.
Mathematical Logic Quarterly,
Vol. 31,
Issue. 21-23,
p.
321.
Simpson, Stephen G.
1985.
Nichtbeweisbarkeit von gewissen kombinatorischen Eigenschaften endlicher Bäume.
Archiv für Mathematische Logik und Grundlagenforschung,
Vol. 25,
Issue. 1,
p.
45.
Drake, F. R.
1985.
How recent work in mathematical logic relates to the foundations of mathematics.
The Mathematical Intelligencer,
Vol. 7,
Issue. 4,
p.
27.
Feferman, Solomon
1985.
Working foundations.
Synthese,
Vol. 62,
Issue. 2,
p.
229.
1985.
Harvey Friedman's Research on the Foundations of Mathematics.
Vol. 117,
Issue. ,
p.
87.
1985.
Harvey Friedman's Research on the Foundations of Mathematics.
Vol. 117,
Issue. ,
p.
137.
1986.
Vol. 122,
Issue. ,
p.
3.
Brown, Douglas K.
and
Simpson, Stephen G.
1986.
Which set existence axioms are needed to prove the separable Hahn-Banach theorem?.
Annals of Pure and Applied Logic,
Vol. 31,
Issue. ,
p.
123.
Simpson, Stephen G.
and
Smith, Rick L.
1986.
Factorization of polynomials and Σ10 induction.
Annals of Pure and Applied Logic,
Vol. 31,
Issue. ,
p.
289.
Harnik, Victor
1987.
Set existence axioms for general (not necessarily countable) stability theory.
Annals of Pure and Applied Logic,
Vol. 34,
Issue. 3,
p.
231.
1989.
Logic Colloquium'87, Proceedings of the Colloquium held in Granada.
Vol. 129,
Issue. ,
p.
11.
Shioji, Naoki
and
Tanaka, Kazuyuki
1990.
Fixed point theory in weak second-order arithmetic.
Annals of Pure and Applied Logic,
Vol. 47,
Issue. 2,
p.
167.
Friedman, Harvey M.
and
Hirst, Jeffry L.
1990.
Weak comparability of well orderings and reverse mathematics.
Annals of Pure and Applied Logic,
Vol. 47,
Issue. 1,
p.
11.
Clark, Peter
1990.
Explanation in Physics: Explanation in Physical Theory.
Royal Institute of Philosophy Supplement,
Vol. 27,
Issue. ,
p.
155.
Yu, Xiaokang
and
Simpson, Stephen G.
1990.
Measure theory and weak König's lemma.
Archive for Mathematical Logic,
Vol. 30,
Issue. 3,
p.
171.
Friedman, Harvey M.
and
Hirst, Jeffry L.
1991.
Reverse mathematics and homeomorphic embeddings.
Annals of Pure and Applied Logic,
Vol. 54,
Issue. 3,
p.
229.
H. Gallier, Jean
1991.
What's so special about Kruskal's theorem and the ordinal Γo? A survey of some results in proof theory.
Annals of Pure and Applied Logic,
Vol. 53,
Issue. 3,
p.
199.
Hirst, Jeffry L.
1992.
Connected components of graphs and reverse mathematics.
Archive for Mathematical Logic,
Vol. 31,
Issue. 3,
p.
183.
Kohlenbach, Ulrich
1993.
Effective moduli from ineffective uniqueness proofs. An unwinding of de La Vallée Poussin's proof for Chebycheff approximation.
Annals of Pure and Applied Logic,
Vol. 64,
Issue. 1,
p.
27.
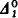 comprehension and
comprehension and 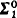 induction. Our main result is that, over RCA0, the Cauchy/Peano Theorem is provably equivalent to weak König's lemma, i.e. the statement that every infinite {0, 1}-tree has a path. We also show that, over RCA0, the Ascoli lemma is provably equivalent to arithmetical comprehension, as is Osgood's theorem on the existence of maximum solutions. At the end of the paper we digress to relate our results to degrees of unsolvability and to computable analysis.
induction. Our main result is that, over RCA0, the Cauchy/Peano Theorem is provably equivalent to weak König's lemma, i.e. the statement that every infinite {0, 1}-tree has a path. We also show that, over RCA0, the Ascoli lemma is provably equivalent to arithmetical comprehension, as is Osgood's theorem on the existence of maximum solutions. At the end of the paper we digress to relate our results to degrees of unsolvability and to computable analysis.
