Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-26T05:53:37.741Z Has data issue: false hasContentIssue false
  • English
  • Français

BETWEEN THE PROBLEMS OF PÓLYA AND TURÁN

Part of: Zeta and $L$-functions: analytic theory Multiplicative number theory Computational number theory

Published online by Cambridge University Press:  27 September 2012

MICHAEL J. MOSSINGHOFF  and
TIMOTHY S. TRUDGIAN
MICHAEL J. MOSSINGHOFF*
Affiliation:
Department of Mathematics, Davidson College, Davidson, NC, 28035-6996, USA (email: mimossinghoff@davidson.edu)
TIMOTHY S. TRUDGIAN
Affiliation:
Department of Mathematics and Computer Science, University of Lethbridge, Alberta, Canada T1K 3M4 (email: tim.trudgian@uleth.ca)
*
✉For correspondence; e-mail: mimossinghoff@davidson.edu
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We investigate the behaviour of the function $L_{\alpha }(x) = \sum _{n\leq x}\lambda (n)/n^{\alpha }$, where 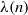$\lambda (n)$ is the Liouville function and $\alpha $ is a real parameter. The case where 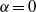$\alpha =0$ was investigated by Pólya; the case 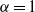$\alpha =1$, by Turán. The question of the existence of sign changes in both of these cases is related to the Riemann hypothesis. 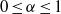Using both analytic and computational methods, we investigate similar problems for the more general family $L_{\alpha }(x)$, where $0\leq \alpha \leq 1$, and their relationship to the Riemann hypothesis and other properties of the zeros of the Riemann zeta function. The case where 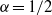$\alpha =1/2$is of particular interest.

Keywords

Liouville functionRiemann hypothesisPólya’s problemTurán’s problem

MSC classification

Secondary: 11M26: Nonreal zeros of $zeta (s)$ and $L(s, chi)$; Riemann and other hypotheses 11N64: Other results on the distribution of values or the characterization of arithmetic functions 11Y35: Analytic computations
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 93 , Issue 1-2 , October 2012 , pp. 157 - 171
Copyright
Copyright © 2012 Australian Mathematical Publishing Association Inc.

Footnotes

Dedicated to the memory of Alf van der Poorten

References

[1]Borwein, P., Ferguson, R. and Mossinghoff, M. J., ‘Sign changes in sums of the Liouville function’, Math. Comp. 77(263) (2008), 1681–1694.CrossRefGoogle Scholar
[2]GMP: The GNU multiple precision arithmetic library. http://gmplib.org.Google Scholar
[3]Haselgrove, C. B., ‘A disproof of a conjecture of Pólya’, Mathematika 5 (1958), 141–145.CrossRefGoogle Scholar
[4]Ingham, A. E., ‘On two conjectures in the theory of numbers’, Amer. J. Math. 64 (1942), 313–319.CrossRefGoogle Scholar
[5]Lehman, R. S., ‘On Liouville’s function’, Math. Comp. 14 (1960), 311–320.Google Scholar
[6]Murty, M. R., Problems in Analytic Number Theory, 2nd edn, Graduate Texts in Mathematics, 206 (Springer, New York, 2008).Google Scholar
[7]Pólya, G., ‘Verschiedene Bemerkungen zur Zahlentheorie’, Jahresber. Deutsch. Math.-Verein. 28 (1919), 31–40.Google Scholar
[8]Rubinstein, M. and Sarnak, P., ‘Chebyshev’s bias’, Experiment. Math. 3(3) (1994), 173–197.CrossRefGoogle Scholar
[9]Tanaka, M., ‘A numerical investigation on cumulative sum of the Liouville function’, Tokyo J. Math. 3(1) (1980), 187–189.CrossRefGoogle Scholar
[10]Titchmarsh, E. C., The Theory of the Riemann Zeta-Function, 2nd edn (Oxford University Press, New York, 1986).Google Scholar
[11]Turán, P., ‘On some approximative Dirichlet-polynomials in the theory of the zeta-function of Riemann’, Danske Vid. Selsk. Mat.-Fys. Medd. 24(17) (1948), 1–36.Google Scholar
[12]Turán, P., ‘Nachtrag zu meiner Abhandlung “On some approximative Dirichlet polynomials in the theory of zeta-function of Riemann”’, Acta Math. Acad. Sci. Hungar. 10 (1959), 277–298.CrossRefGoogle Scholar