Article contents
DISTALITY OF CERTAIN ACTIONS ON 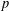 $p$-ADIC SPHERES
$p$-ADIC SPHERES
Published online by Cambridge University Press: 29 July 2019
Abstract
Consider the action of 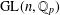 $\operatorname{GL}(n,\mathbb{Q}_{p})$ on the
$\operatorname{GL}(n,\mathbb{Q}_{p})$ on the 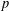 $p$-adic unit sphere
$p$-adic unit sphere  ${\mathcal{S}}_{n}$ arising from the linear action on
${\mathcal{S}}_{n}$ arising from the linear action on  $\mathbb{Q}_{p}^{n}\setminus \{0\}$. We show that for the action of a semigroup
$\mathbb{Q}_{p}^{n}\setminus \{0\}$. We show that for the action of a semigroup  $\mathfrak{S}$ of
$\mathfrak{S}$ of 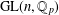 $\operatorname{GL}(n,\mathbb{Q}_{p})$ on
$\operatorname{GL}(n,\mathbb{Q}_{p})$ on  ${\mathcal{S}}_{n}$, the following are equivalent: (1)
${\mathcal{S}}_{n}$, the following are equivalent: (1) 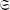 $\mathfrak{S}$ acts distally on
$\mathfrak{S}$ acts distally on  ${\mathcal{S}}_{n}$; (2) the closure of the image of
${\mathcal{S}}_{n}$; (2) the closure of the image of 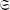 $\mathfrak{S}$ in
$\mathfrak{S}$ in  $\operatorname{PGL}(n,\mathbb{Q}_{p})$ is a compact group. On
$\operatorname{PGL}(n,\mathbb{Q}_{p})$ is a compact group. On  ${\mathcal{S}}_{n}$, we consider the ‘affine’ maps
${\mathcal{S}}_{n}$, we consider the ‘affine’ maps 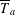 $\overline{T}_{a}$ corresponding to
$\overline{T}_{a}$ corresponding to 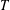 $T$ in
$T$ in  $\operatorname{GL}(n,\mathbb{Q}_{p})$ and a nonzero
$\operatorname{GL}(n,\mathbb{Q}_{p})$ and a nonzero  $a$ in
$a$ in  $\mathbb{Q}_{p}^{n}$ satisfying
$\mathbb{Q}_{p}^{n}$ satisfying 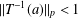 $\Vert T^{-1}(a)\Vert _{p}<1$. We show that there exists a compact open subgroup
$\Vert T^{-1}(a)\Vert _{p}<1$. We show that there exists a compact open subgroup 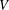 $V$, which depends on
$V$, which depends on 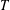 $T$, such that
$T$, such that 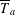 $\overline{T}_{a}$ is distal for every nonzero
$\overline{T}_{a}$ is distal for every nonzero 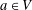 $a\in V$ if and only if
$a\in V$ if and only if  $T$ acts distally on
$T$ acts distally on 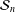 ${\mathcal{S}}_{n}$. The dynamics of ‘affine’ maps on
${\mathcal{S}}_{n}$. The dynamics of ‘affine’ maps on 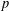 $p$-adic unit spheres is quite different from that on the real unit spheres.
$p$-adic unit spheres is quite different from that on the real unit spheres.
Information
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 109 , Issue 2 , October 2020 , pp. 250 - 261
- Copyright
- © 2019 Australian Mathematical Publishing Association Inc.
Footnotes
R. Shah would like to acknowledge the support of DST (SERB) through the MATRICS research grant. A. K. Yadav would like to acknowledge the support for a research assistantship from DST-PURSE grant in Jawaharlal Nehru University.
References
- 3
- Cited by


