No CrossRef data available.
Published online by Cambridge University Press: 19 August 2013
The aim of the present paper is to extend the dualizing object approach to Stone duality to the noncommutative setting of skew Boolean algebras. This continues the study of noncommutative generalizations of different forms of Stone duality initiated in recent papers by Bauer and Cvetko-Vah, Lawson, Lawson and Lenz, Resende, and also the current author. In this paper we construct a series of dual adjunctions between the categories of left-handed skew Boolean algebras and Boolean spaces, the unital versions of which are induced by dualizing objects 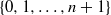 $\{ 0, 1, \ldots , n+ 1\} $,
$\{ 0, 1, \ldots , n+ 1\} $, 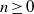 $n\geq 0$. We describe the categories of Eilenberg-Moore algebras of the monads of the adjunctions and construct easily understood noncommutative reflections of left-handed skew Boolean algebras, where the latter can be faithfully embedded (if
$n\geq 0$. We describe the categories of Eilenberg-Moore algebras of the monads of the adjunctions and construct easily understood noncommutative reflections of left-handed skew Boolean algebras, where the latter can be faithfully embedded (if 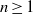 $n\geq 1$) in a canonical way. As an application, we answer the question that arose in a recent paper by Leech and Spinks to describe the left adjoint to their ‘twisted product’ functor
$n\geq 1$) in a canonical way. As an application, we answer the question that arose in a recent paper by Leech and Spinks to describe the left adjoint to their ‘twisted product’ functor 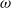 $\omega $.
$\omega $.