EXPLICIT INTERPOLATION BOUNDS BETWEEN HARDY SPACE AND 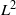${L}^{2} $

Abstract

Every bounded linear operator that maps 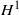${H}^{1} $ to 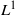${L}^{1} $ and 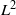${L}^{2} $ to 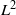${L}^{2} $ is bounded from 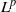${L}^{p} $ to 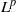${L}^{p} $ for each 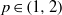$p\in (1, 2)$, by a famous interpolation result of Fefferman and Stein. We prove 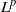${L}^{p} $-norm bounds that grow like $O(1/ (p- 1))$ as 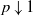$p\downarrow 1$. This growth rate is optimal, and improves significantly on the previously known exponential bound 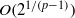$O({2}^{1/ (p- 1)} )$. For 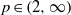$p\in (2, \infty )$, we prove explicit 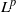${L}^{p} $ estimates on each bounded linear operator mapping 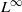${L}^{\infty } $ to bounded mean oscillation (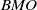$\mathit{BMO}$) and 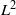${L}^{2} $ to 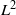${L}^{2} $. This 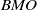$\mathit{BMO}$ interpolation result implies the 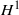${H}^{1} $ result above, by duality. In addition, we obtain stronger results by working with dyadic 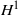${H}^{1} $ and dyadic 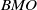$\mathit{BMO}$. The proofs proceed by complex interpolation, after we develop an optimal dyadic ‘good lambda’ inequality for the dyadic $\sharp $-maximal operator.