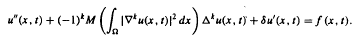Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Jung, Il-Hyo
and
Choi, Jong-Sool
2006.
ENERGY DECAY ESTIMATES FOR A KIRCHHOFF MODEL WITH VISCOSITY.
Bulletin of the Korean Mathematical Society,
Vol. 43,
Issue. 2,
p.
245.
Kim, Daewook
Kim, Dojin
Hong, Keum-Shik
and
Jung, Il Hyo
2014.
Global Existence and Energy Decay Rates for a Kirchhoff-Type Wave Equation with Nonlinear Dissipation.
The Scientific World Journal,
Vol. 2014,
Issue. ,
p.
1.


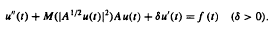 .
.