Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-27T05:48:39.805Z Has data issue: false hasContentIssue false
  • English
  • Français

Ideals of compact operators

Part of: Linear spaces and algebras of operators Normed linear spaces and Banach spaces; Banach lattices

Published online by Cambridge University Press:  09 April 2009

Åsvald Lima  and
Eve Oja
Åsvald Lima
Affiliation:
Department of Mathematics, Agder College, Gimlemoen 25J, Serviceboks 422, 4604 Kristiansand, Norway e-mail: asvald.Lima@hia.no
Eve Oja
Affiliation:
Faculty of Mathematics, Tartu University, Liivi 2-606, EE-50409 Tartu, Estonia e-mail: eveoja@math.ut.ee
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We give an example of a Banach space X such that K (X, X) is not an ideal in K (X, X**). We prove that if z* is a weak* denting point in the unit ball of Z* and if X is a closed subspace of a Banach space Y, then the set of norm-preserving extensions H B(x* ⊗ z*) ⊆ 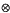(Z*, Y)* of a functional x* ⊗ Z* ∈ (Z ⊗ X)* is equal to the set H B(x*) ⊗ {z*}. Using this result, we show that if X is an M-ideal in Y and Z is a reflexive Banach space, then K (Z, X) is an M-ideal in K(Z, Y) whenever K (Z, X) is an ideal in K (Z, Y). We also show that K (Z, X) is an ideal (respectively, an M-ideal) in K (Z, Y) for all Banach spaces Z whenever X is an ideal (respectively, an M-ideal) in Y and X * has the compact approximation property with conjugate operators.

Keywords

compact operatoridealM-idealapproximation property

MSC classification

Secondary: 46B20: Geometry and structure of normed linear spaces 46B28: Spaces of operators; tensor products; approximation properties 47L05: Linear spaces of operators
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 77 , Issue 1 , August 2004 , pp. 91 - 110
Copyright
Copyright © Australian Mathematical Society 2004

References

[1]Alfsen, E. M. and Effros, E. G., ‘Structure in real Banach spaces. Part I and II’, Ann. of Math. 96 (1972), 98–173.CrossRefGoogle Scholar
[2]Cabello, C. J., Nieto, E. and Oja, E., ‘On ideals of compact operators satisfying the M(r, s)-inequality’, J. Math. Anal. Appl. 220 (1998), 334–348.CrossRefGoogle Scholar
[3]Casazza, P. G. and Jarchow, H., ‘Self-induced compactness in Banach spaces’, Proc. Royal Soc. Edinburgh Sect. A 126 (1996), 355–362.CrossRefGoogle Scholar
[4]Casazza, P. G. and Kalton, N. J., ‘Notes on approximation properties in separable Banach spaces’, in: Geometry of Banach Spaces, Proc. Conf. Strobl 1989(eds. Müller, P. F. X. and Schachermayer, W.), London Math. Soc. Lecture Note Series 158 (Cambridge University Press, 1990) pp. 49–63.Google Scholar
[5]Davis, W. J., Figiel, T., Johnson, W. B. and Pelczyński, A., ‘Factoring weakly compact operators’, J. Funct. Analysis 17 (1974), 311–327.CrossRefGoogle Scholar
[6]Diestel, J. and Uhl, J. J. Jr, Vector measures, Mathematical Surveys 15 (Amer. Math. Soc., Providence, RI, 1977).CrossRefGoogle Scholar
[7]Feder, M. and Saphar, P. D., ‘Spaces of compact operators and their dual spaces’, Israel J. Math. 21 (1975), 38–49.CrossRefGoogle Scholar
[8]Godefroy, G., Kalton, N. J. and Saphar, P. D., ‘Unconditional ideals in Banach spaces’, Studia Math. 104 (1993), 13–59.CrossRefGoogle Scholar
[9]Godefroy, G. and Saphar, P. D., ‘Normes lisses et propriété d'approximation métrique’, C. R. Acad. Sci. Paris. Sér. l. 299 (1984), 753–756.Google Scholar
[10]Godefroy, G. and Saphar, P. D., ‘Duality in spaces of operators and smooth norms on Banach spaces’, Illinois J. Math. 32 (1988), 672–695.CrossRefGoogle Scholar
[11]Harmand, P., Werner, D. and Werner, W., M-ideals in Banach spaces and Banach algebras, Lecture Notes in Math. 1547 (Springer, Berlin, 1993).CrossRefGoogle Scholar
[12]Johnson, J., ‘Remarks on Banach spaces of compact operators’, J. Funct. Analysis 32 (1979), 304–311.CrossRefGoogle Scholar
[13]Johnson, W. B. and Oikhberg, T., ‘Separable lifting property and extensions of local reflexivity’, Illinois J. Math. 45 (2001), 123–137.CrossRefGoogle Scholar
[14]Lima, Å., ‘Intersection properties of balls and subspaces in Banach spaces’, Trans. Amer. Math. Soc. 227 (1977), 1–62.CrossRefGoogle Scholar
[15]Lima, Å., ‘The metric approximation property, norm-one projections and intersection properties of balls’, Israel J. Math. 84 (1993), 451–475.CrossRefGoogle Scholar
[16]Lima, Å., ‘Property (wM*) and the unconditional metric compact approximation property’, Studia Math. 113 (1995), 249–263.CrossRefGoogle Scholar
[17]Lima, Å., Nygaard, O. and Oja, E., ‘Isometric factorization of weakly compact operators and the approximation property’, Israel J. Math. 119 (2000), 325–348.CrossRefGoogle Scholar
[18]Lima, Å. and Oja, E., ‘Ideals of finite rank operators, intersection properties of balls, and the approximation property’, Studia Math. 133 (1999), 175–186.Google Scholar
[19]Lima, Å. and Oja, E., ‘Hahn-Banach extension operators and spaces of operators’, Proc. Amer. Math. Soc. 130 (2002), 3631–3640.CrossRefGoogle Scholar
[20]Lima, Å., Oja, E., Rao, T. S. S. R. K. and Werner, D., ‘Geometry of operator spaces’, Michigan Math. J. 41 (1994), 473–490.CrossRefGoogle Scholar
[21]Lindenstrauss, J. and Tzafriri, L., Classical Banach spaces l, Ergebnisse der Mathematik und ihrer Grenzgebiete 92 (Springer, 1977).CrossRefGoogle Scholar
[22]Oja, E., ‘HB-subspaces and Godun sets of subspaces in Banach spaces’, Mathematika 44 (1997), 120–132.CrossRefGoogle Scholar
[23]Oja, E., ‘Géométrie des espaces de Banach ayant des approximations de l'identité contractantes’, C. R.Acad. Sci. Paris, Sér. I 328 (1999), 1167–1170.Google Scholar
[24]Oja, E., ‘Geometry of Banach spaces having shrinking approximations of the identity’, Trans. Amer. Math. Soc. 352 (2000), 2801–2823.CrossRefGoogle Scholar
[25]Oja, E. and Põldvere, M., ‘On subspaces of Banach spaces where every functional has a unique norm-preserving extension’, Studia Math. 117 (1996), 289–306.Google Scholar
[26]Oja, E. and Põldvere, M., ‘Intersection properties of ball sequences and uniqueness of Hahn-Banach extensions’, Proc. Royal Soc. Edinburgh 129 A (1999), 1251–1262.CrossRefGoogle Scholar
[27]Phelps, R. R., ‘Uniqueness of Hahn-Banach extensions and unique best approximation’, Trans. Amer. Math. Soc. 95 (1960), 238–255.Google Scholar
[28]Rao, T. S. S. R. K., ‘On ideals in Banach spaces’, Rocky Mountain J. Math. 31 (2001), 595–609.CrossRefGoogle Scholar
[29]Sims, B. and Yost, D., ‘Linear Hahn-Banach extension operators’, Proc. Edinburgh Math. Soc. 32 (1989), 53–57.CrossRefGoogle Scholar
[30]Werner, D., ‘Denting points in tensor products of Banach spaces’, Proc. Amer. Math. Soc. 101 (1987), 122–126.CrossRefGoogle Scholar
[31]Werner, D., ‘M-Structure in tensor products of Banach spaces’, Math. Scand. 61 (1987), 149–164.CrossRefGoogle Scholar
[32]Willis, G., ‘The compact approximation property does not imply the approximation property’, Studia Math. 103 (1992), 99–108.CrossRefGoogle Scholar