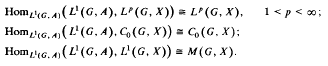Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Quek, T.S
1986.
Multipliers of certain vector valued function spaces.
Journal of Mathematical Analysis and Applications,
Vol. 115,
Issue. 2,
p.
406.
Lai, Hang-Chin
and
Chang, Tsu-Kung
1988.
Proceedings of the Analysis Conference, Singapore 1986.
Vol. 150,
Issue. ,
p.
151.
Lai, Hang-Chin
and
Chang, Tsu Kung
1989.
Multipliers and translation invariant operators.
Tohoku Mathematical Journal,
Vol. 41,
Issue. 1,
Haro, J.C. Candeal
and
Lai, H.C.
1992.
Multipliers in continuous vector-valued function spaces.
Bulletin of the Australian Mathematical Society,
Vol. 46,
Issue. 2,
p.
199.
Haro, J. C. Candeal
and
Lai, H. C.
1995.
Multipliers in vector-valued function spaces under convolution.
Acta Mathematica Hungarica,
Vol. 67,
Issue. 3,
p.
175.
Öztop, Serap
2003.
A note on multipliers of Lp(G, A).
Journal of the Australian Mathematical Society,
Vol. 74,
Issue. 1,
p.
25.
Swain, Jitendriya
and
Ramakrishnan, Radha
2010.
L p -multipliers for the Hilbert space valued functions on the Heisenberg group.
Monatshefte für Mathematik,
Vol. 160,
Issue. 1,
p.
95.
Khan, Liaqat Ali
and
Alsulami, Saud M.
2014.
Multipliers of Modules of Continuous Vector-Valued Functions.
Abstract and Applied Analysis,
Vol. 2014,
Issue. ,
p.
1.