Hostname: page-component-745bb68f8f-cphqk Total loading time: 0 Render date: 2025-01-13T13:37:47.223Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE RANKIN–SELBERG ZETA FUNCTION

Part of: Multiplicative number theory Real functions Integral transforms, operational calculus

Published online by Cambridge University Press:  19 September 2012

ALEKSANDAR IVIĆ
ALEKSANDAR IVIĆ*
Affiliation:
Katedra Matematike RGF-a, Universitet u Beogradu, Đušina 7, 11000 Beograd, Serbia (email: ivic@rgf.bg.ac.rs, aivic@matf.bg.ac.rs)
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We obtain the approximate functional equation for the Rankin–Selberg zeta function in the critical strip and, in particular, on the critical line 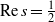$\operatorname {Re} s= \frac {1}{2}$.

Keywords

Rankin–Selberg zeta functionapproximate functional equation

MSC classification

Secondary: 11N37: Asymptotic results on arithmetic functions 44A15: Special transforms (Legendre, Hilbert, etc.) 26A12: Rate of growth of functions, orders of infinity, slowly varying functions
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 93 , Issue 1-2 , October 2012 , pp. 101 - 113
Copyright
Copyright © 2012 Australian Mathematical Publishing Association Inc.

References

[1]Deligne, P., ‘La conjecture de Weil’, Publ. Math. Inst. Hautes Études Sci. 43 (1974), 273–307.CrossRefGoogle Scholar
[2]Erdélyi, A., Magnus, W., Oberhettinger, F. and Tricomi, F. G., Higher Transcendental Functions. Volume I (McGraw-Hill, New York, 1953).Google Scholar
[3]Hardy, G. H. and Littlewood, J. E., ‘The approximate functional equation for $\zeta (s)$ and $\zeta ^2(s)$’, Proc. Lond. Math. Soc. 29 (1929), 81–97.CrossRefGoogle Scholar
[4]Huxley, M. N., ‘Exponential sums and the Riemann zeta function V’, Proc. Lond. Math. Soc. 90 (2005), 1–41.CrossRefGoogle Scholar
[5]Ivić, A., The Riemann Zeta-Function (Wiley, New York, 1985), (2nd edn, Dover, Mineola, NY, 2003).Google Scholar
[6]Ivić, A., Lectures on Mean Values of the Riemann Zeta-Function, Lectures on Mathematics and Physics, 82 (Springer, Berlin, 1991).Google Scholar
[7]Ivić, A., ‘An approximate functional equation for a class of Dirichlet series’, J. Anal. (Madras, India) 3 (1995), 241–252.Google Scholar
[8]Ivić, A., Matsumoto, K. and Tanigawa, Y., ‘On Riesz mean of the coefficients of the Rankin–Selberg series’, Math. Proc. Cambridge Philos. Soc. 127 (1999), 117–131.CrossRefGoogle Scholar
[9]Kaczorowski, A. and Perelli, A., ‘The Selberg class: a survey’, in: Number Theory in Progress, Proc. Conf. in Honour of A. Schinzel, (eds. Győry, K., Iwaniec, H. and Urbanowicz, J.) (W. de Gruyter, Berlin, 1999), pp. 953–992.CrossRefGoogle Scholar
[10]Li, H. and Wu, J., ‘The universality of symmetric power $L$-functions and their Rankin–Selberg $L$-functions’, J. Math. Soc. Japan 59 (2007), 371–392.CrossRefGoogle Scholar
[11]Rankin, R. A., ‘Contributions to the theory of Ramanujan’s function $\tau (n)$ and similar arithmetical functions II. The order of the Fourier coefficients of integral modular forms’, Proc. Cambridge Philos. Soc. 35 (1939), 357–372.CrossRefGoogle Scholar
[12]Rankin, R. A., Modular Forms and Functions (Cambridge University Press, Cambridge, 1977).CrossRefGoogle Scholar
[13]Sankaranarayanan, A., ‘Fundamental properties of symmetric square $L$-functions I’, Illinois J. Math. 46 (2002), 23–43.CrossRefGoogle Scholar
[14]Selberg, A., ‘Bemerkungen über eine Dirichletsche Reihe, die mit der Theorie der Modulformen nahe verbunden ist’, Arch. Math. Naturvid. 43 (1940), 47–50.Google Scholar
[15]Selberg, A., Old and new conjectures and results about a class of Dirichlet series’, Proc. Amalfi Conf. Analytic Number Theory 1989 (eds. E. Bombieri et al.), University of Salerno, Salerno, 1992, pp. 367–385.Google Scholar
[16]Shimura, G., ‘On the holomorphy of certain Dirichlet series’, Proc. Lond. Math. Soc. 31 (1975), 79–98.CrossRefGoogle Scholar
[17]Wiebelitz, R., ‘Über approximative Funktionalgleichungen der Potenzen der Riemannschen Zeta-funktion’, Math. Nachr. 6 (1951–1952), 263–270.CrossRefGoogle Scholar