Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-28T01:58:08.115Z Has data issue: false hasContentIssue false
  • English
  • Français

ON VON NEUMANN–JORDAN CONSTANTS

Part of: Normed linear spaces and Banach spaces; Banach lattices

Published online by Cambridge University Press:  15 December 2009

KAZUO HASHIMOTO  and
GEN NAKAMURA
KAZUO HASHIMOTO*
Affiliation:
Hiroshima Jogakuin University, 4-13-1 Ushita Higashi Higashi-ku, Hiroshima 732-0063, Japan (email: hasimoto@gaines.hju.ac.jp)
GEN NAKAMURA
Affiliation:
Matsue College of Technology, 14-4 Nishi-ikuma, Matsue, Shimane 690-8518, Japan (email: nakamura@matsue-ct.ac.jp)
*
✉For correspondence; e-mail: hasimoto@gaines.hju.ac.jp
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

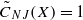In this note, we provide an example of a Banach space X for which that is not isomorphic to any Hilbert space, where denotes the infimum of all von Neumann–Jordan constants for equivalent norms of X.

Keywords

von Neumann–Jordan constantnth von Neumann–Jordan constantHilbert spaceisomorphism

MSC classification

Secondary: 46B03: Isomorphic theory (including renorming) of Banach spaces
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 87 , Issue 3 , December 2009 , pp. 371 - 375
Copyright
Copyright © Australian Mathematical Publishing Association, Inc. 2009

References

[1]Clarkson, J. A., ‘The von Neumann–Jordan constant for the Lebesgue space’, Ann. of Math. (2) 38 (1937), 114–115.CrossRefGoogle Scholar
[2]Jordan, P. and von Neumann, J., ‘On inner products in linear metric spaces’, Ann. of Math. (2) 36 (1935), 719–723.CrossRefGoogle Scholar
[3]Kato, M., Maligranda, L. and Takahashi, Y., ‘On James and Jordan–von Neumann constants and normal structure coefficient of Banach spaces’, Studia Math. 144(2) (2001), 275–295.CrossRefGoogle Scholar
[4]Kato, M. and Takahashi, Y., ‘Uniform convexity, uniform non-squareness and von Neumann–Jordan constant for Banach spaces’, RIMS Kokyuroku 939 (1996), 87–96.Google Scholar
[5]Kato, M. and Takahashi, Y., ‘On the von Neumann–Jordan constant for Banach spaces’, Proc. Amer. Math. Soc. 125 (1997), 1055–1062.CrossRefGoogle Scholar
[6]Kato, M. and Takahashi, Y., ‘Von Neumann–Jordan constant for Lebesgue–Bochner spaces’, J. Inequal. Appl. 2 (1998), 89–97.Google Scholar
[7]Kato, M., Takahashi, Y. and Hashimoto, K., ‘On n-th von Neumann–Jordan constants for Banach spaces’, Bull. Kyushu Inst. Technol. Pure Appl. Math. 45 (1998), 25–33.Google Scholar
[8]Takahashi, Y. and Kato, M., ‘Von Neumann–Jordan constant and uniformly non-square Banach spaces’, Nihonkai Math. J. 9 (1998), 155–169.Google Scholar