Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-26T07:12:35.329Z Has data issue: false hasContentIssue false
  • English
  • Français

Oscillatory and asymptotic properties of homogeneous and nonhomogeneous delay differential equations of even order

Published online by Cambridge University Press:  09 April 2009

Raymond D. Terry
Raymond D. Terry
Affiliation:
California Polytechnic State University, San Luis Obispo, California, U.S.A.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we consider the (non)oscillation properties of two general nonhomogeneous nonlinear delay differential equations of order 2n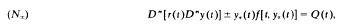 using as background and motivation the techniques previously applied to the associated homogeneous delay differential equations H+ and H−. The equations N+ and N− are each reduced to homogeneous form by the introduction of transformations u(t) = y(t) – R(t) and v(t) = R(t) — y(t), where R(t) is a solution of the associated nonhomogeneous differential equation (N). We first extend certain results for the equation H+ and then develop a classification of the positive solutions of equation H−. Using this classification and the one developed by Terry (1974) for H+ we develop a natural classification of the positive solutions of N+ and N− according to the sign properties of the derivatives of u(t) and v(t). For each choice of R(t), it is seen that there are 2n + 1 types of positive solutions of N+or N–. An intermediate Riccati transformation is employed to obtain integral criteriafor the nonexistence of some of these solutions. Analysis of the Taylor remainder results in sufficient conditions for the nonexistence of other such solutions.

Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 22 , Issue 3 , November 1976 , pp. 282 - 304
Copyright
Copyright © Australian Mathematical Society 1976

References

Burton, L. P. (1952), ‘Oscillation theorems for the solutions of linear, nonhomogeneous, secondorder differential systems’, Pacific J. Math. 2, 281–289.CrossRefGoogle Scholar
Howard, H. C. (to appear), ‘Oscillation and nonoscillation criteria for nonhomogeneous differential equations.’Google Scholar
Kartsatos, A. G. (1971), ‘On the maintenance of oscillations under the effect of a small forcing term’, J. Differential Equations 10, 355–363.CrossRefGoogle Scholar
Kartsatos, A. G. (1972), ‘Maintenance of oscillations under the effect of a periodic forcing term’, Proc. Amer. Math. Soc. 33, 377–383.CrossRefGoogle Scholar
Kartsatos, A. G. and Manougian, M. M. (to appear), ‘Perturbations causing oscillations of functional differential equations’, Proc. Amer. Math. Soc.Google Scholar
Kusano, T. (to appear), ‘Differential equations with retarded arguments‘, Proc. Caratheodory Symposium, Athens, Greece (1973).Google Scholar
Kusano, T. and Onose, H. (1974), ‘Oscillations of functional differential equations with retarded argument’, J. Differential Equations 15, 269–277.CrossRefGoogle Scholar
Ladas, G. (1971a), ‘Oscillation and asymptotic behavior of solutions of differential equations with retarded arguments’, J. Differential Equations 10, 281–290.CrossRefGoogle Scholar
Ladas, G. (1971b), ‘On principal solutions of nonlinear differential equations’, J. Math. Anal. Appl. 36, 103–109.CrossRefGoogle Scholar
Singh, B. (to appear), ‘Asymptotically vanishing oscillatory trajectories in second order retarded equations’, Siam J. Math. Anal.Google Scholar
Singh, B. and Dahiya, R. S. (to appear), ‘Effect of delays on oscillation in functional differential equations with time lag’, Siam J. Appl. Math.Google Scholar
Terry, R. D. (1974), ‘Oscillatory properties of a delay differential equation of even order’, Pacific J. Math. 52, 269–282.CrossRefGoogle Scholar
Terry, R. D. and Wong, P. K. (1972), ‘Oscillatory properties of a fourth-order delay differential equation’, Funkcial. Ekvac. 15, 209–221.Google Scholar
Wong, P. K. (1969), ‘On a class of nonlinear fourth order differential equations’, Ann. Mat. Pura Appl. 81, 331–346.CrossRefGoogle Scholar