No CrossRef data available.
Article contents
Polynomial approximations on a polydisc1
Published online by Cambridge University Press: 09 April 2009
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Throughout this paper, we will use the terminologies and notations as in [4]. Thus, UN denotes the open unit polydisc in the space CN of N complex variables, TN the distinguished boundary of UN and 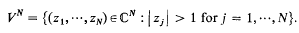 .
.
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 14 , Issue 2 , September 1972 , pp. 216 - 218
- Copyright
- Copyright © Australian Mathematical Society 1972
References
[1]Chui, C. K., ‘Bounded approximation by polynomials whose zeros lie on a circle’, Trans. Amer. Math. Soc. 138 (1969), 171–182.CrossRefGoogle Scholar
[2]Chui, C. K., ‘C-polynomial approximation of Hp and ℋp functions’, J. Math. Analysis and Appl. To appear.Google Scholar
[3]Korevaar, J., ‘Approximation by polynomials whose zeros lie on a circle’, Nieuw Arch. Wisk (3) 10 (1962), 11–16.Google Scholar


