Hostname: page-component-78c5997874-j824f Total loading time: 0 Render date: 2024-11-13T05:44:27.775Z Has data issue: false hasContentIssue false
  • English
  • Français

Toeplitz operators on certain weakly pseudoconvex domains

Part of: Special classes of linear operators Selfadjoint operator algebras

Published online by Cambridge University Press:  09 April 2009

David Crocker  and
Iain Raeburn
David Crocker
Affiliation:
School of Mathematics, University of New South Wales, Post Office Box 1, Kensington, N.S.W. 2033, Australia
Iain Raeburn
Affiliation:
School of Mathematics, University of New South Wales, Post Office Box 1, Kensington, N.S.W. 2033, Australia
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let Ω be the weakly pseudoconvex domain

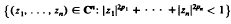
and let ∂Ω be its boundary. If ϕ ∈ L∞ (∂Ω), we denote by Tϕ, the Toephtz operator with symbol ϕ acting on the Hardy space H2(∂Ω), and by J(∂Ω) the C*-subalgebra of B(H2(∂Ω)) generated by the Toeplitz operators with continuous symbol. Our main theorem asserts that J(∂Ω) contains the ideal K of all compact operators on H2(∂Ω), and that the symbol map ϕ→Tϕ induces an isomorphism of C(∂Ω) onto the quotient C*-algebra ℑ(∂Ω)/K. Similar results have been established before for other domains, and in particular when Ω is strongly pseudoconvex. The main interest of our results lies in their proofs: ours are elementary, whereas those used in the strongly pseudoconvex case depend heavily on the theory of the tangential Cauchy-Riemann operator.

MSC classification

Secondary: 47B35: Toeplitz operators, Hankel operators, Wiener-Hopf operators 46L05: General theory of $C^*$-algebras
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 31 , Issue 1 , June 1981 , pp. 1 - 14
Copyright
Copyright © Australian Mathematical Society 1981

References

de Monvel, L. Boutet (1979), ‘On the index of Toeplitz operators of several complex variables’, Invent. Math. 50, 249–272.CrossRefGoogle Scholar
Coburn, L. A. (1973), ‘Singular integral operators and Toeplitz operators on odd spheres’, Indiana Univ. Math. J. 23, 433–439.Google Scholar
D'Angelo, J. P. (1978), ‘A note on the Bergmann kernel’, Duke Math. J. 45, 259–265.Google Scholar
Douglas, R. G. (1972a), Banach algebra techniques in operator theory (Academic Press, New York).Google Scholar
Douglas, R. G. (1972b), Banach algebra techniques in the theory of Toeplitz operators (CMBS regional conference series in mathematics 15, Amer. Math. Soc., Providence).Google Scholar
Erdélyi, A. (Editor) (1953), Higher transcendental functions, Vol. 1 (McGraw-Hill, New York).Google Scholar
Folland, G. B. and Kohn, J. J. (1972), The Neumann problem for the Cauchy-Riemann complex (Annals of Mathematics studies 75, Princeton University Press, Princeton, N. J.).Google Scholar
Hakim, M. et Sibony, N. (1977), ‘Quelques conditions pour l'existence de fonctions pics dans des domaines pseudoconvexes’, Duke Math. J. 44, 399–406.Google Scholar
Janas, J. (1976a), ‘Index formula for Toeplitz operators in certain strongly pseudoconvex domains’, Bull. Acad. Polon. Sci. Ser. Sci. Math. Astronom. Phys. 24, 433–436.Google Scholar
Janas, J. (1976b), ‘An application of the theorem of Rudin to the Toeplitz operators on odd spheres’, Math. Z. 150, 185–187.Google Scholar
Jewell, N. P. and Krantz, S. G. (1979), ‘Toeplitz operators and related function algebras on certain pseudoconvex domains’, Trans. Amer. Math. Soc. 252, 297–312.Google Scholar
Raeburn, I. (1978), ‘On Toeplitz operators associated with strongly pseudoconvex domains’, Studia Math. 63, 253–258.Google Scholar
Sato, H. and Yabuta, K. (1978); ‘Toeplitz operators on strongly pseudoconvex domains in Stein spaces’, Tôhoku Math. J. 30, 153–162.CrossRefGoogle Scholar
Venugopalkrishna, U. (1972), ‘Fredholm operators associated with strongly pseudoconvex domains’, J. Functional Analysis 9, 349–373.Google Scholar
Webster, S. M. (1979), ‘Biholomorphic mappings and the Bergmann kernel off the diagonal’, Invent. Math. 51, 155–169.Google Scholar