Hostname: page-component-5cf477f64f-fcbfl Total loading time: 0 Render date: 2025-03-25T12:25:55.859Z Has data issue: false hasContentIssue false
  • English
  • Français

UNBOUNDED DERIVATIONS IN ALGEBRAS ASSOCIATED WITH MONOTHETIC GROUPS

Part of: Selfadjoint operator algebras

Published online by Cambridge University Press:  13 January 2020

SLAWOMIR KLIMEK  and
MATT McBRIDE
SLAWOMIR KLIMEK
Affiliation:
Department of Mathematical Sciences, Indiana University-Purdue University Indianapolis, 402 N. Blackford St., Indianapolis, IN 46202, USA e-mail: sklimek@math.iupui.edu
MATT McBRIDE*
Affiliation:
Department of Mathematics and Statistics, Mississippi State University, 175 President’s Cir., Mississippi State, MS 39762, USA
*
e-mail: mmcbride@math.msstate.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

Given an infinite, compact, monothetic group 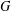$G$ we study decompositions and structure of unbounded derivations in a crossed product 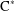$\text{C}^{\ast }$-algebra 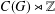$C(G)\rtimes \mathbb{Z}$ obtained from a translation on $G$ by a generator of a dense cyclic subgroup. We also study derivations in a Toeplitz extension of the crossed product and the question whether unbounded derivations can be lifted from one algebra to the other.

Keywords

derivationsoperator algebraMonothetic groups

MSC classification

Primary: 46L52: Noncommutative function spaces
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 111 , Issue 3 , December 2021 , pp. 345 - 371
Check for updates
Copyright
© 2020 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Communicated by L. O. Clark

References

Bratteli, O., Derivations, Dissipations and Group Actions on C∗ -algebras, Lecture Notes in Mathematics, 1229 (Springer, Berlin–Heidelberg, 1986).10.1007/BFb0098817CrossRefGoogle Scholar
Bratteli, O., Elliott, G. A. and Jorgensen, P. E. T., ‘Decomposition of unbounded derivations into invariant and approximately inner parts’, J. reine angew. Math. 346 (1984), 166–193.Google Scholar
Downarowicz, T., ‘Survey of odometers and Toeplitz flows’, Contemp. Math. 385 (2005), 7–37.10.1090/conm/385/07188CrossRefGoogle Scholar
Fillmore, P., A User’s Guide to Operator Algebras (Wiley-Interscience, Toronto, 1996).Google Scholar
Halmos, P. and Samelson, H., ‘On monothetic groups’, Proc. Amer. Math. Soc. 28 (1942), 254–258.Google ScholarPubMed
Katok, A. and Hasselblatt, B., Introduction to the Modern Theory of Dynamical Systems (Cambridge University Press, Cambridge, 1996).Google Scholar
Klimek, S., McBride, M. and Rathnayake, S., ‘Derivations and spectral triples on quantum domains II: quantum annulus’, Sci. China Math. (2018), doi:10.1007/s11425-018-9317-3.Google Scholar
Klimek, S., McBride, M., Rathnayake, S. and Sakai, K., ‘The quantum pair of pants’ SIGMA, 11 (to appear). Published online (10 February 2015).10.3842/SIGMA.2015.012CrossRefGoogle Scholar
Klimek, S., McBride, M., Rathnayake, S., Sakai, K. and Wang, H., ‘Derivations and spectral triples on quantum domains I: quantum disk’, SIGMA 13(075) (2017), 1–26.Google Scholar
Klimek, S., McBride, M., Rathnayake, S., Sakai, K. and Wang, H., ‘Unbounded derivations in Bunce–Deddens–Toeplitz algebras’, J. Math. Anal. Appl. 15 (2019), 988–1020.10.1016/j.jmaa.2019.02.001CrossRefGoogle Scholar
Kurka, P., Topological and Symbolic Dynamics (Société Mathématique de France, Paris, 2003).Google Scholar
Morris, S., Pontryagin Duality and the Structure of Locally Compact Abelian Groups (Cambridge University Press, Cambridge, 1977).10.1017/CBO9780511600722CrossRefGoogle Scholar
Pedersen, G. K., ‘Lifting derivations from quotients of separable C∗ -algebras’, Proc. Natl. Acad. Sci. USA 73 (1976), 1414–1415.10.1073/pnas.73.5.1414CrossRefGoogle Scholar
Robert, A., A Course in p-adic Analysis (Springer, New York, 2000).10.1007/978-1-4757-3254-2CrossRefGoogle Scholar
Sakai, S., Operator Algebras in Dynamical Systems (Cambridge University Press, Cambridge, 1991).10.1017/CBO9780511662218CrossRefGoogle Scholar
Willard, S., General Topology (Addison-Wesley, Reading, MA, 1970).Google Scholar