Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-11T07:27:51.615Z Has data issue: false hasContentIssue false
  • English
  • Français

Varieties of distributive lattices with unary operations I

Part of: Distributive lattices Algebraic logic Varieties Boolean algebras (Boolean rings)

Published online by Cambridge University Press:  09 April 2009

H. A. Priestley
H. A. Priestley
Affiliation:
Mathematical Institute24/29 St Giles Oxford OX1 3LBEngland e-mail: hap@maths.ox.ac.uk
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A unified study is undertaken of finitely generated varieties HSP (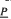) of distributive lattices with unary operations, extending work of Cornish. The generating algebra (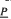) is assusmed to be of the form (P; ∧, ∨, 0, 1, {fμ}), where each fμ is an endomorphism or dual endomorphism of (P; ∧, ∨, 0, 1), and the Priestly dual of this lattice is an ordered semigroup N whose elements act by left multiplication to give the maps dual to the operations fμ. Duality theory is fully developed within this framework, into which fit many varieties arising in algebraic logic. Conditions on N are given for the natural and Priestley dualities for HSP (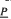) to be essentially the same, so that, inter alia, coproducts in HSP (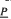) are enriched D-coproducts.

Keywords

Priestley dualitynatural dualityfree algebramonoid.

MSC classification

Secondary: 06D05: Structure and representation theory 06D25: Post algebras 03G20: Łukasiewicz and Post algebras 08B99: None of the above, but in this section
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 63 , Issue 2 , October 1997 , pp. 165 - 207
Copyright
Copyright © Australian Mathematical Society 1997

References

[1]Adams, M. E. and Priestley, H. A., ‘Equational bases for varieties of Ockham algebras’, Algebra Universalis 32 (1994), 368–397.CrossRefGoogle Scholar
[2]Balbes, R. and Dwinger, Ph., Distributive lattices (University of Missouri Press, Columbia, 1974).Google Scholar
[3]Blyth, T. S., Noor, A. S. A. and Varlet, J. C., ‘Ockham algebras with de Morgan skeletons’, J. Algebra 117 (1988), 165–178.CrossRefGoogle Scholar
[4]Blyth, T. S., Noor, A. S. A. and Varlet, J. C., ‘Equational bases for subvarieties of double MS-algebras’, Glasgow Math. J. 31 (1989), 1–16.Google Scholar
[5]Blyth, T. S. and Varlet, J. C., ‘subdirectly irreducible double MS-algebras’, Proc. Roy. Soc. Edinburgh Sect. A 98 (1994), 241–247.Google Scholar
[6]Blyth, T. S. and Varlet, J. C., Ockham algebras (Oxford University Press, Oxford, 1994).CrossRefGoogle Scholar
[7]Burris, S. and Sankappanavar, H. P., A course in universal algebra (Springer, New York, 1981).CrossRefGoogle Scholar
[8]Cignoli, R. and de Gallego, M. S., ‘The lattice structure of some Łukasiewicz algebras’, Algebra Universalis 13 (1981), 315–328.CrossRefGoogle Scholar
[9]Cignoli, R. and de Gallego, M. S., ‘Dualities for some De Morgan algebras with operators and Łukasiewicz algebras’, J. Austral. Math. Soc. (Series A) 34 (1983), 377–393.CrossRefGoogle Scholar
[10]Clark, D. M. and Davey, B. A., Natural dualities for the working algebraist, in preparation (Cambridge University Press).Google Scholar
[11]Cornish, W. H., ‘Monoids acting on distributive lattices’, manuscript (invited talk at the annual meeting of the Austral. Math. Soc., May 1977).Google Scholar
[12;]Cornish, W. H., Antimorphic action. Categories of algebraic structures with involutions or antiendomorphisms, R & E Res. Exp. Math., 12 (Heldermann Verlag, Berlin, 1986).Google Scholar
[13]Cornish, W. H. and Fowler, P. R., ‘Coproducts of Kleene algebras’, J. Austral. Math. Soc. (Series A) 27 (1979), 209–220.CrossRefGoogle Scholar
[14]Davey, B. A., ‘On the lattice of subvarieties’, Houston J. Math. 5 (1979), 183–192.Google Scholar
[15]Davey, B. A., ‘Duality theory on ten dollars a day’, in: Algebras and orders (eds. Rosenberg, I. G. and Sabidussi, G.) NATO Advanced Study Institute Series, Series C, 389 (Kluwer, Dordrecht, 1993), 71–111.Google Scholar
[16]Davey, B. A. and Priestley, H. A., ‘Lattices of homomorphisms’, J. Austral. Math. Soc. (Series A) 40 (1986), 364–406.CrossRefGoogle Scholar
[17]Davey, B. A. and Priestley, H. A., ‘Generalised piggyback dualities and applications to Ockham algebras’, Houston J. Math. 13 (1987), 151–197.Google Scholar
[18]Davey, B. A. and Priestley, H. A., Introduction to lattices and order (Cambridge University Press, Cambridge, 1990).Google Scholar
[19]Davey, B. A. and Werner, H., ‘Dualities and equivalences for varieties of algebras’, in: Contributions to lattice theory (Szeged, 1980) (eds. Huhn, A. P. and Schmidt, E. T.) Colloq. Math. Soc. János Bolyai 33, (North-Holland, Amsterdam 1983), pp. 101–275.Google Scholar
[20]Davey, B. A. and Werner, H., ‘Piggyback-dualitäten’, Bull. Austral. Math. Soc. 32 (1985), 1–32.CrossRefGoogle Scholar
[21]Davey, B. A. and Werner, H., ‘Piggyback dualities’, in: Colloq. Math. Soc. Jhnos Bolyai 43 (1986), 61–83.Google Scholar
[22]Goldberg, M. S., ‘Distributive Ockham algebras: free algebras and injectivity’, Bull. Austral. Math. Soc. 24 (1981), 161–203.Google Scholar
[23]Guzman, F. and Squier, C. C., ‘Subdirectly irreducible and free Kleene-Stone algebras’, Algebra Universalis 31 (1994), 266–273.CrossRefGoogle Scholar
[24]Priestley, H. A., ‘Ordered sets and duality for distributive lattices’, in: Orders, description and roles (eds. Pouzet, M. and Richard, D.), Ann. Discrete Math. 23 (North-Holland, Amsterdam, 1984), pp. 39–60.Google Scholar
[25]Priestley, H. A., ‘The determination of the lattices of subvarieties of certain congruence-distributive varieties’, Algebra Universalis 32 (1994), 44–62.CrossRefGoogle Scholar
[26]Priestley, H. A., ‘Natural dualities’, in: Lattice theory and its applications—a volume in honor of Garrett Birkhoff's 80th birthday (eds. Baker, K. A. and Wille, R.) (Heldermann Verlag, Berlin, 1995), pp. 185–209.Google Scholar
[27]Priestley, H. A., ‘A note on equational bases’, Bull. Soc. Roy. Sci. Liège 64 (1995), 113–118.Google Scholar
[28]Priestley, H. A., ‘Natural dualities for varieties of n-valued Lukasiewicz algebras’, Studia Logica 54, 333–370.CrossRefGoogle Scholar
[29]Priestley, H. A., ‘Dualities and identities I’, preprint.Google Scholar
[30]Priestley, H. A. and Santos, R., ‘Dualities and identities II: DMS-algebras’, preprint.Google Scholar
[31]Priestley, H. A. and Santos, R., ‘Varieties of distributive lattices with unary operations II’, Portugal Math., to appear.Google Scholar
[32]Santos, R., ‘Involutive Stone algebras and regular α De Morgan algebras’, manuscript.Google Scholar
[33]Santos, R., ‘Natural dualities for some subvarieties of DMS’, manuscript.Google Scholar
[34]Sankappanavar, H. P., ‘Linked double weak Stone algebras’, Z. Math. Logik Grundlag. Math. 35 (1989), 485–494.CrossRefGoogle Scholar
[35]Sequeira, M., ‘Double MS n-algebras and double K m.n-algebras’, Glasgow Math. J. 35 (1993), 189–201.Google Scholar
[36]Urquhart, A., ‘Lattices with a dual homomorphic operation’, Studia Logica 38 (1979), 201–209.CrossRefGoogle Scholar
[37]Urquhart, A., ‘Lattices with a dual homomorphic operation II’, Studia Logica 40 (1981), 391–404.Google Scholar
[38]Varlet, J. C., ‘MS-algebras: a survey’, in: Lattices, semigroups and universal algebra (Lisbon, 1988) (eds. Almeida, J., Bordalo, G. and Dwinger, Ph.) (Plenum Press, New York, 1990), pp. 299–313.CrossRefGoogle Scholar