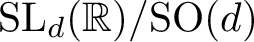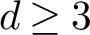Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Humphries, Peter
and
Thorner, Jesse
2025.
New Variants of Arithmetic Quantum Ergodicity.
Communications in Mathematical Physics,
Vol. 406,
Issue. 3,