INTÉGRALES ORBITALES SUR $GL(N,\mathbb{F}_{q}((t)))$

Résumé

Soit $F$ un corps local non archimédien de caractéristique 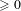${\geqslant}0$, et soit 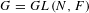$G=GL(N,F)$, 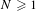$N\geqslant 1$. Un élément 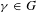$\unicode[STIX]{x1D6FE}\in G$ est dit quasi régulier si le centralisateur de $\unicode[STIX]{x1D6FE}$ dans 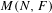$M(N,F)$ est un produit d’extensions de $F$. Soit 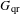$G_{\text{qr}}$ l’ensemble des éléments quasi réguliers de $G$. Pour 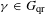$\unicode[STIX]{x1D6FE}\in G_{\text{qr}}$, on note 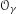$O_{\unicode[STIX]{x1D6FE}}$ l’intégrale orbitale ordinaire sur 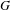$G$ associée à $\unicode[STIX]{x1D6FE}$. On remplace ici le discriminant de Weyl 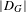$|D_{G}|$ par un facteur de normalisation 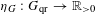$\unicode[STIX]{x1D702}_{G}:G_{\text{qr}}\rightarrow \mathbb{R}_{{>}0}$ permettant d’obtenir les mêmes résultats que ceux prouvés par Harish-Chandra en caractéristique nulle: pour 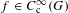$f\in C_{\text{c}}^{\infty }(G)$, l’intégrale orbitale normalisée 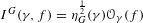$I^{G}(\unicode[STIX]{x1D6FE},f)=\unicode[STIX]{x1D702}_{G}^{\frac{1}{2}}(\unicode[STIX]{x1D6FE})O_{\unicode[STIX]{x1D6FE}}(f)$ est bornée sur 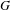$G$, et pour 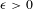$\unicode[STIX]{x1D716}>0$ tel que 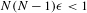$N(N-1)\unicode[STIX]{x1D716}<1$, la fonction 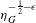$\unicode[STIX]{x1D702}_{G}^{-\frac{1}{2}-\unicode[STIX]{x1D716}}$ est localement intégrable sur 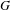$G$.

Abstract

Let $F$ be a non–Archimedean local field of characteristic 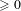${\geqslant}0$, and let 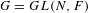$G=GL(N,F)$, 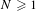$N\geqslant 1$. An element 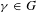$\unicode[STIX]{x1D6FE}\in G$ is said to be quasi–regular if the centralizer of $\unicode[STIX]{x1D6FE}$ in 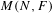$M(N,F)$ is a product of field extensions of $F$. Let 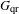$G_{\text{qr}}$ be the set of quasi–regular elements of 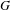$G$. For $\unicode[STIX]{x1D6FE}\in G_{\text{qr}}$, we denote by 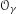$O_{\unicode[STIX]{x1D6FE}}$ the ordinary orbital integral on 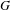$G$ associated with $\unicode[STIX]{x1D6FE}$. In this paper, we replace the Weyl discriminant $|D_{G}|$ by a normalization factor 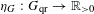$\unicode[STIX]{x1D702}_{G}:G_{\text{qr}}\rightarrow \mathbb{R}_{{>}0}$ which allows us to obtain the same results as proven by Harish–Chandra in characteristic zero: for $f\in C_{\text{c}}^{\infty }(G)$, the normalized orbital integral 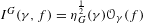$I^{G}(\unicode[STIX]{x1D6FE},f)=\unicode[STIX]{x1D702}_{G}^{\frac{1}{2}}(\unicode[STIX]{x1D6FE})O_{\unicode[STIX]{x1D6FE}}(f)$ is bounded on 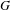$G$, and for 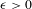$\unicode[STIX]{x1D716}>0$ such that 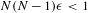$N(N-1)\unicode[STIX]{x1D716}<1$, the function $\unicode[STIX]{x1D702}_{G}^{-\frac{1}{2}-\unicode[STIX]{x1D716}}$ is locally integrable on 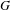$G$.