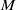Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Schapira, Pierre
2018.
Algebraic and Analytic Microlocal Analysis.
Vol. 269,
Issue. ,
p.
63.
Ike, Yuichi
Matsui, Yutaka
and
Takeuchi, Kiyoshi
2018.
Hyperbolic localization and Lefschetz fixed point formulas for higher-dimensional fixed point sets.
International Mathematics Research Notices,
Vol. 2018,
Issue. 15,
p.
4852.