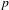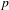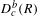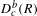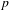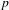Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
SUZUKI, TAKASHI
2020.
DUALITY FOR COHOMOLOGY OF CURVES WITH COEFFICIENTS IN ABELIAN VARIETIES.
Nagoya Mathematical Journal,
Vol. 240,
Issue. ,
p.
42.
Lai, King-Fai
Longhi, Ignazio
Suzuki, Takashi
Tan, Ki-Seng
and
Trihan, Fabien
2021.
On the μ-invariants of abelian varieties over function fields of positive characteristic.
Algebra & Number Theory,
Vol. 15,
Issue. 4,
p.
863.
Trihan, Fabien
and
Brinon, Olivier
2022.
On the zeroes and poles of L-functions over varieties in positive characteristic.
Journal für die reine und angewandte Mathematik (Crelles Journal),
Vol. 2022,
Issue. 789,
p.
265.