Article contents
Random Dieudonné modules, random 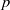 $p$-divisible groups, and random curves over finite fields
$p$-divisible groups, and random curves over finite fields
Published online by Cambridge University Press: 13 February 2013
Abstract
We describe a probability distribution on isomorphism classes of principally quasi-polarized 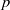 $p$-divisible groups over a finite field
$p$-divisible groups over a finite field  $k$ of characteristic
$k$ of characteristic  $p$ which can reasonably be thought of as a ‘uniform distribution’, and we compute the distribution of various statistics (
$p$ which can reasonably be thought of as a ‘uniform distribution’, and we compute the distribution of various statistics (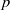 $p$-corank,
$p$-corank,  $a$-number, etc.) of
$a$-number, etc.) of 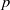 $p$-divisible groups drawn from this distribution. It is then natural to ask to what extent the
$p$-divisible groups drawn from this distribution. It is then natural to ask to what extent the 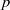 $p$-divisible groups attached to a randomly chosen hyperelliptic curve (respectively, curve; respectively, abelian variety) over
$p$-divisible groups attached to a randomly chosen hyperelliptic curve (respectively, curve; respectively, abelian variety) over 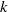 $k$ are uniformly distributed in this sense. This heuristic is analogous to conjectures of Cohen–Lenstra type for
$k$ are uniformly distributed in this sense. This heuristic is analogous to conjectures of Cohen–Lenstra type for 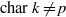 $\text{char~} k\not = p$, in which case the random
$\text{char~} k\not = p$, in which case the random 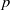 $p$-divisible group is defined by a random matrix recording the action of Frobenius. Extensive numerical investigation reveals some cases of agreement with the heuristic and some interesting discrepancies. For example, plane curves over
$p$-divisible group is defined by a random matrix recording the action of Frobenius. Extensive numerical investigation reveals some cases of agreement with the heuristic and some interesting discrepancies. For example, plane curves over  ${\mathbf{F} }_{3} $ appear substantially less likely to be ordinary than hyperelliptic curves over
${\mathbf{F} }_{3} $ appear substantially less likely to be ordinary than hyperelliptic curves over 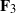 ${\mathbf{F} }_{3} $.
${\mathbf{F} }_{3} $.
MSC classification
Information
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 12 , Issue 3 , July 2013 , pp. 651 - 676
- Copyright
- ©Cambridge University Press 2013
References
- 7
- Cited by

